Numbers – Definition, Types, Examples, Practice Problems, FAQs

What Are Numbers?

A number is an arithmetic value used to represent quantity. Hence, a number is a mathematical concept used to count, measure, and label. Thus, numbers form the basis of mathematics.

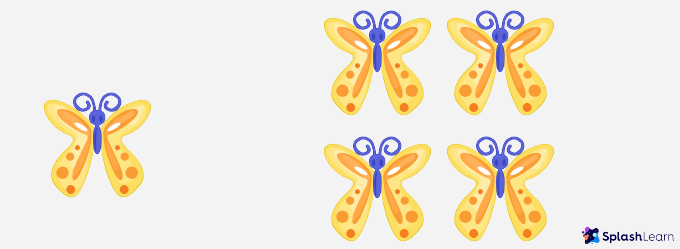
For example, this is 1 butterfly and these are 4 butterflies.

History of Numbers

The inscriptions found at archaeological sites show that early humans used various symbols to show numbers. For example, ancient farmers, traders, and merchants used tally marks to show quantities. In tally marks, a standing line is drawn for each count and the fifth count is shown by striking off the four lines. This, however, was a tedious way and it was not feasible to show quantities.

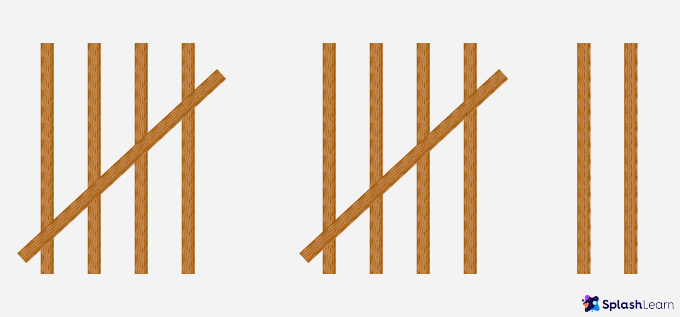
Different ways of writing numbers were brought to use with the development of early civilizations. They used different symbols to show larger quantities. But even with these systems, it was not easy to show large quantities.

Around the seventh century, a decimal (or base ten) positional method, was perfected in India. This method used ten unique symbols to represent any number or quantity. These symbols are 0, 1, 2, 3, 4, 5 6, 7, 8, and 9.

This system was spread across Europe by the Arab merchants, scholars, and conquerors.

This system is called the Hindu–Arabic numeral system, and it remains the most common system for representing numbers to date.

Numbers in Everyday Life

Numbers are used everywhere around us. Your birthday has numbers that tell the day, month, and year you were born. 

Numbers are used to keep track of time. We use clocks that show us time. We plan our day and events according to time. 

Numbers are involved in buying and selling too. To count money and the units of an item, we use numbers. 

Numbers are used for measurement. Temperature, weight, length, capacity, speed, distance, area, volume, and so on are measured using numbers. 

Numbers play an important role in our body too. We have 2 eyes, 2 ears, 1 nose, 2 hands, 2 legs, and an adult body has 206 bones. 

Our houses have numbers, bank accounts have numbers, and so do our cars, buses, trains, and flights.

Number Representation

1. Digits 0-9

A number system is a writing system for denoting numbers using digits or symbols in a logical manner.

We use the digits from 0 to 9 to form all other numbers.

With the help of these digits, we can create infinite numbers.

For example, 121; 34,987; 2,987,633; 459,227,904; …

This number system using 10 digits is called the Decimal Number System.

2. Alphabetical Form of Number

Number words are the alphabetical form of numbers. As the name suggests, these are numbers written in words.

For example:

1  One

2 Two

15 Fifteen

33 Thirty-three 

3. Symbolically, Using Numerals

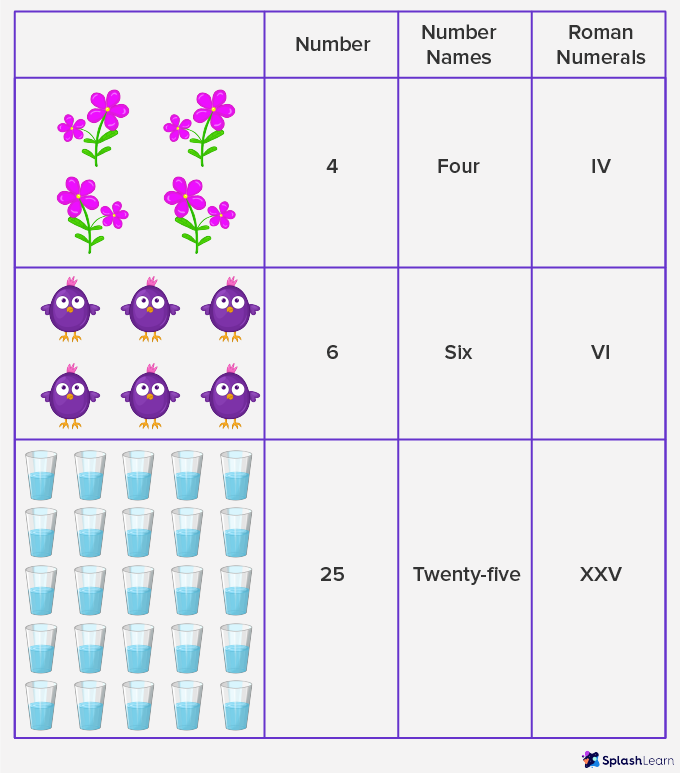
Numerical symbols are numerals, such as Hindu-Arabic numerals (for example: 112, 415, 999) or Roman numerals (I, II, V, VIII). 

Cardinal Numbers and Ordinal Numbers

Cardinal numbers are counting numbers. The numbers that we use for counting are called cardinal numbers.

Cardinal numbers tell us how many things, items, or objects are there.

Example: 1, 2, 3, 10, 158

Ordinal numbers give us the exact position of a thing, item, or an object in the list. Ordinal numbers tell the position of an object rather than its quantity. 

Example: 1st, 2nd, 3rd, 9th, 150th 

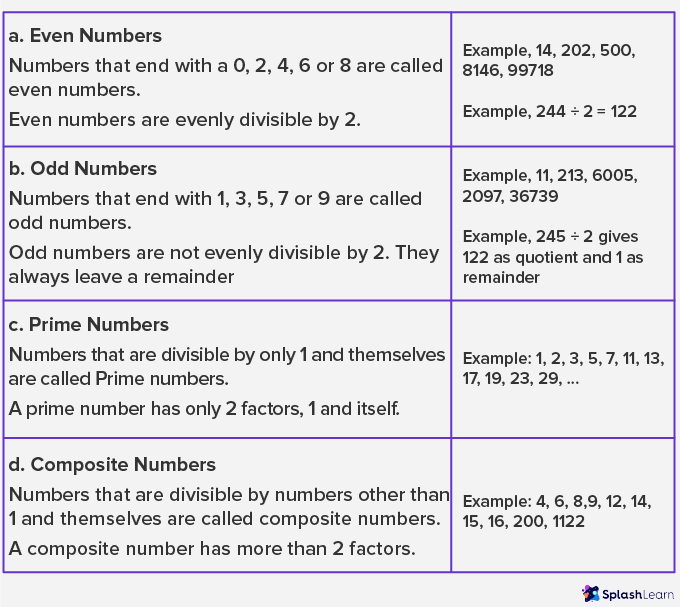
Types of Numbers

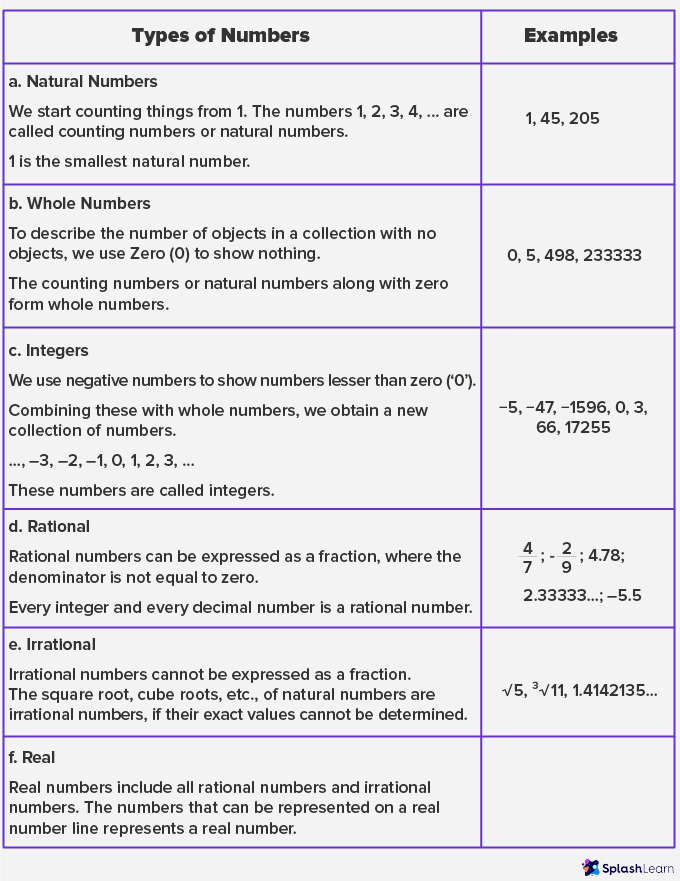
Apart from the above, there exist other numbers, namely even and odd numbers, prime numbers, and composite numbers. These can be defined as follows:

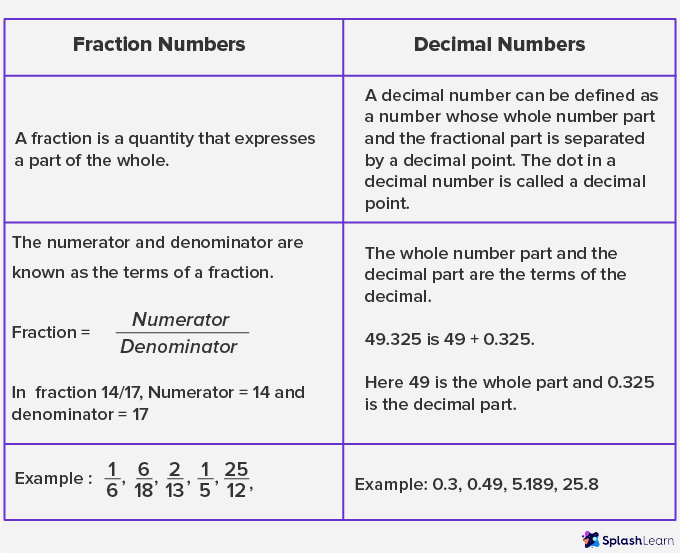
Fraction and Decimal Numbers:

Solved Examples on Numbers

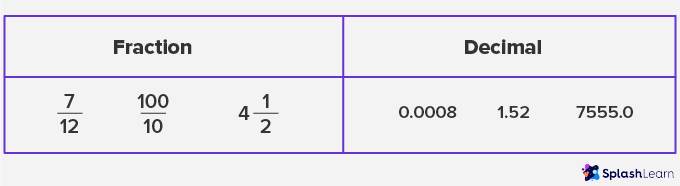
Example 1: Classify the given set of numbers as fractions or decimals.

7/12; 0.0008; 1.52; 100/10; 4 1/2; 7555.0

Solution:

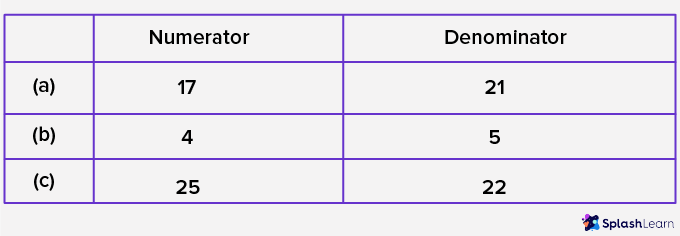
Example 2: Write the numerator and denominator of given rational numbers.

1. 17/21 (b) 4/5 (c) 25/22

Solution:

Example 3: Write the numbers in words.

1. 548
2. 1,660

Solution:

1. Five hundred and forty-eight.
2. One thousand six hundred and sixty.

Practice Problems On Numbers

Frequently Asked Questions On Numbers