Isosceles Right Triangle: Definition with Examples

What Is an Isosceles Right Triangle?

An isosceles right triangle is a right-angled triangle whose base and height (legs) are equal in length. It is a type of special isosceles triangle where one interior angle is a right angle and the remaining two angles are thus congruent since the angles opposite to the equal sides are equal. 

It is also known by the name of right-angled isosceles triangle or a right isosceles triangle. 

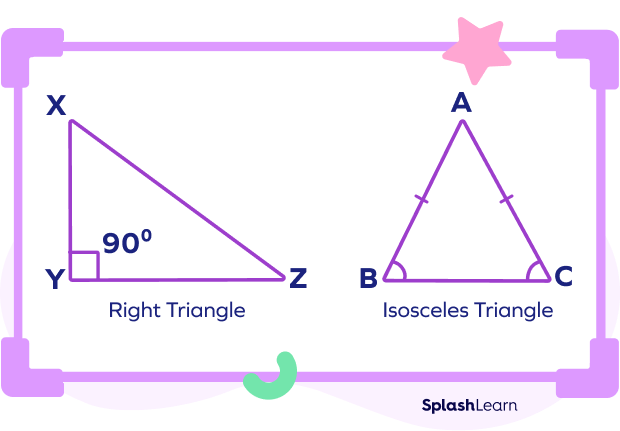
When you combine these two properties together, you get an isosceles right triangle.

Isosceles Right Triangle: Definition

An isosceles right triangle is a type of right triangle whose legs (base and height) are equal in length. 

Since the two sides of the right triangle are equal in measure, the corresponding angles are also equal. Therefore, in an isosceles right triangle, two sides and the two acute angles are equal.

Thus, the interior angles of an isosceles right triangle are $90^{\circ},\; 45^{\circ},\;45^{\circ}$.

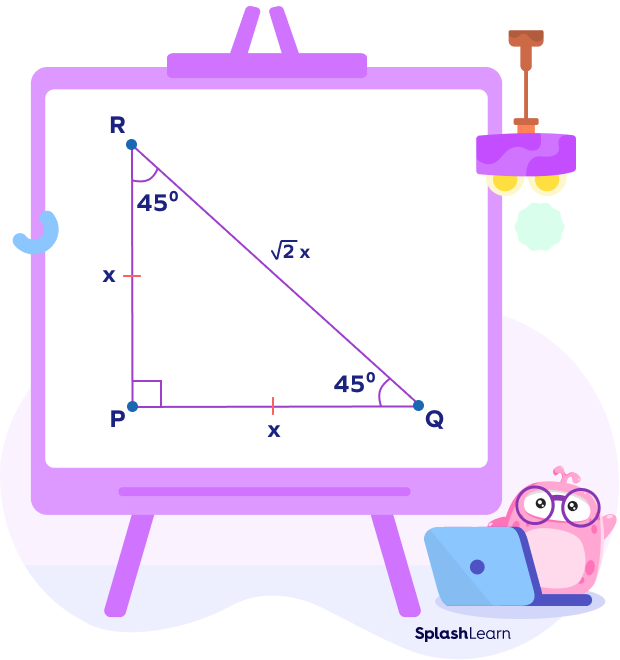
Isosceles Right Triangle Hypotenuse

The hypotenuse of a right angled triangle is the longest side of the triangle, which is opposite to the right angle. 

It is equal to exactly $√2$ times the length of the congruent sides of the triangle. If the length of each of the equal sides is x units, then the length of the hypotenuse of the isosceles right triangle will be equal to $\sqrt{2x}$ units. 

This property is derived using the Pythagoras’ theorem. Here’s a right triangle, $\Delta PQR$: 

How to Find the Hypotenuse of an Isosceles Triangle

To find the hypotenuse of an isosceles right triangle, we use the Pythagorean theorem.

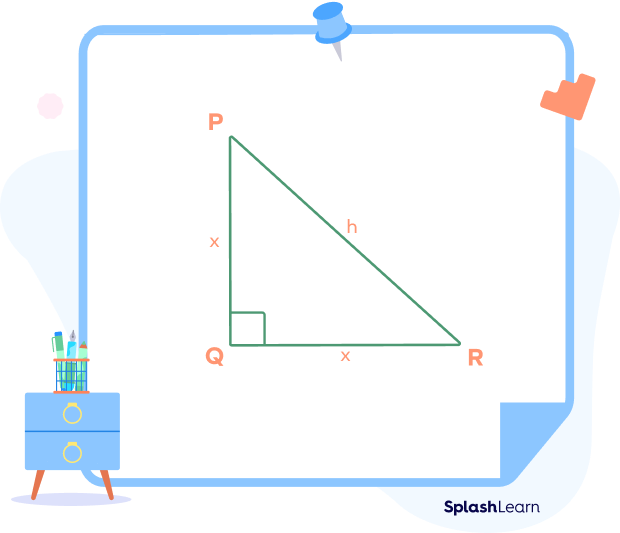
We know that in an isosceles right triangle, two sides are of equal length.

Now, if we assume both the sides to be equal to x units, the hypotenuse can be calculated as:

$Hypotenuse^{2} = x^{2} + x^{2}$ _______________(By Pythagoras’ theorem)

$Hypotenuse^{2} = 2x^{2}$

$Hypotenuse= \sqrt{2x^{2}}$

$Hypotenuse= \sqrt{2}x$

We can use the above derived result as it is without applying the Pythagoras’ theorem every time. The length of the hypotenuse of an isosceles right triangle will always be equal to $√2$ times the length of the equal sides of the triangle.

Isosceles Right Triangle Formulas

We just discussed the formula to find the hypotenuse of an isosceles right triangle. It is given by

Hypotenuse $= \sqrt{2} \times$ Length of congruent sides

Now let’s discuss the formulas to find the perimeter and area of the right angled isosceles triangle.

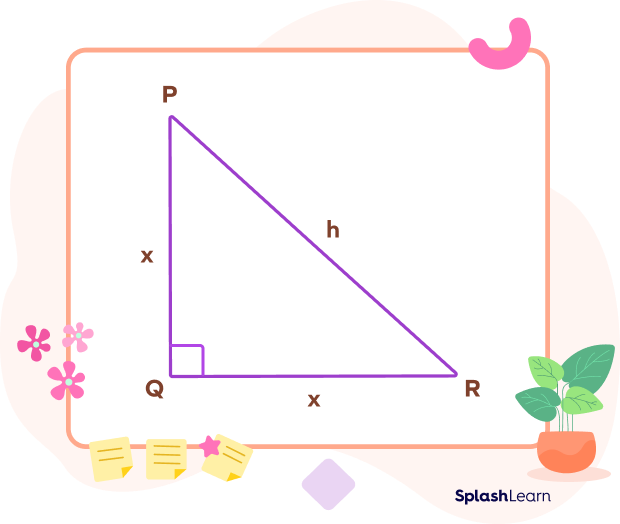
Area of an Isosceles Right Triangle

Area of a triangle $= \frac{1}{2} \times$ Base $\times$ Height. 

In $\Delta PQR ,\; PQ = QR = x$, where PQ is the height and QR is the base. 

The area of the isosceles right triangle $= \frac{1}{2} \times PQ \times QR$

  $= \frac{x \times x}{2}$ 

   $= \frac{x^{2}}{2}$ sq. units.

Therefore, the area of the isosceles right triangle formula is $\frac{x^{2}}{2}$, where “x” is equal to the congruent side length.

Perimeter of an Isosceles Right Triangle

Perimeter of a triangle $=$ Sum of lengths of all its sides

Perimeter of isosceles right triangle $\Delta PQR = PQ + QR + PR$

$PQ = QR = x$ units 

$PR = h$ units

Perimeter of the isosceles right triangle formula $= x + x + h = (2x + h)$ units.

Therefore, the perimeter of the isosceles right triangle formula is $2x + h$, where “x” represents the length of congruent sides and “h” is equal to the length of the hypotenuse.

Isosceles Right Triangle Properties

1. It has one interior angle, which is equal to $90^{\circ}$.
2. The sides or legs are perpendicular to each other and are also known as the base and the height. They are congruent.
3. The other two angles of an isosceles right triangle are acute angles and are congruent to each other. They are equal to $45^{\circ}$ each.
4. The sum of all the interior angles measures $180^{\circ}$.
5. If we draw an altitude from the right angle vertex to the hypotenuse (the opposite side), then it is the perpendicular bisector of the hypotenuse. 
6. The area of an isosceles right triangle is equal to $12 \times$ Base $\times$ Height square units.

Fun Facts!

• Since the two sides of an isosceles right triangle are equal, the angles are also equal. And both the angles measure 45 degrees.
• Right angle is always the largest angle in a right triangle.
• There can’t be any obtuse angles in a right triangle.

Conclusion

In this article, we explored the properties of the isosceles right triangle. Let’s solve some practice problems for better understanding.

Solved Examples on Isosceles Right

1. The equal sides of an isosceles right triangle are 5 units each. Calculate the length of its hypotenuse.

Solution:

We know that the formula to calculate the hypotenuse of an isosceles right triangle is:

Hypotenuse $= \sqrt{2\; x}$

where, “x” is the length of the congruent side.

The equal sides measure 5 units.

Therefore, the hypotenuse will be equal to $\sqrt{2 \times 5} = 7.071$ units.

2. The length of the base of an isosceles right triangle is 10 units. What will be the area of this triangle?

Solution:

We know that the formula to calculate the area of an isosceles right triangle is:

$\frac{x^{2}}{2}$ square units, where x is the measure of the congruent side of the triangle.

Area $= \frac{10^{2}}{2} = \frac{100}{2} = 50$ square units.

3. The area of an isosceles right triangle is 72 square units. What is the measure of its base?

Solution:

We know that the formula to calculate the area of an isosceles right triangle is:

$\frac{x^{2}}{2}$ square units, where x is the measure of the congruent side of the triangle.

Given that the area of the triangle is 72 square units.

Putting this value in the formula:

$\frac{x^{2}}{2} = 72$

$x^{2} = 72 \times 2 = 144$

$x = \sqrt{144}$

$x = 12$ units.

Therefore, the measure of the base of an isosceles right triangle with area equal to 72 square units is 12 units.

4. The height of a right isosceles triangle is 8 units. What will be its perimeter?

Solution:

The perimeter of an isosceles right triangle $= (2x + h)$ units.

x is equal to the length of the congruent side

h is the measure of the hypotenuse.

The height of this isosceles right triangle is 8 units.

Here,

$Hypotenuse = \sqrt{2x}$

$h = \sqrt{2 \times 8} = 11.3137$ units

$Perimeter = 2x + h = (2 \times 8) + 11.3137 = 27.3137$ units.

5. The perimeter of an isosceles right triangle is equal to $10 + 5\sqrt{2}$ units. If the non-congruent side is equal to $5\sqrt{2}$ units then, find the measure of the congruent sides. 

Solution:

We know that the formula to calculate the perimeter of an isosceles right triangle is: $(2x + h)$ units.

Where, x is equal to the length of the congruent side and h is the measure of the hypotenuse.

$Perimeter = 10 + 5\sqrt{2}$ units

Hypotenuse $= 5\sqrt{2}$ units

Putting this in the above formula:

$10 + 5\sqrt{2} = 2x + 5\sqrt{2}$

$10 = 2x$

$x = 5$ units.

Therefore, the congruent side is equal to 5 units.

Practice Problems on Isosceles Right Triangle

Frequently Asked Questions on Isosceles Right Triangle