- What Is Mixed Numbers to Improper Fractions Conversion?
- How to Convert a Mixed Number to an Improper Fraction
- Mixed Number to Improper Fraction Conversion Chart
- Solved Examples on Mixed Number to Improper Fraction Conversion
- Practice Problems on Mixed Number to Improper Fraction Conversion
- Frequently Asked Questions on Mixed Number to Improper Fraction Conversion
What Is Mixed Numbers to Improper Fractions Conversion?
A whole number and a proper fraction together create a mixed number. An improper fraction is a fraction in which the numerator is greater than the denominator.
For instance, $\frac{2}{3}$ is a proper fraction since its denominator is bigger than its numerator, but $\frac{3}{2}$ is an improper fraction since “numerator $\gt$ denominator.”

Any mixed number can be written in its improper fraction form and vice versa since they both basically represent the same fraction, just written in different forms. How can we turn a mixed number into an improper fraction? We will learn in this article!
Mixed Fractions
Mixed fraction is composed of a whole number and a proper fraction.
Example: $2\frac{1}{7}$ is a mixed fraction, where 2 is the whole number part and $\frac{1}{7}$ is a proper fraction. This mixed number (or mixed fraction) lies between 2 and 3.
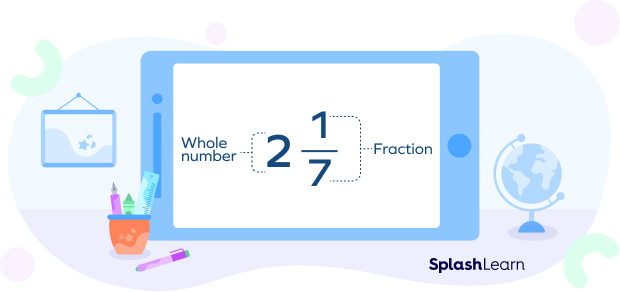
Improper Fraction
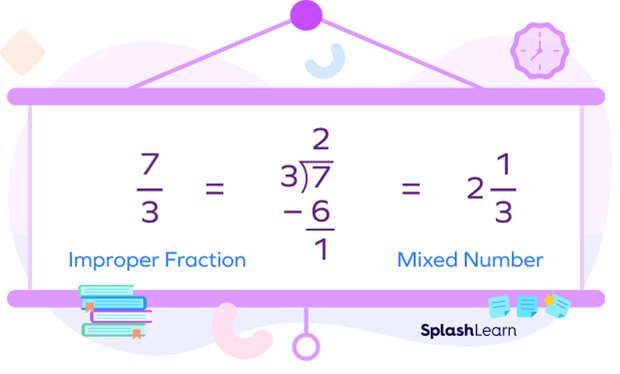
A fraction that has the numerator higher than or equal to the denominator is said to be inappropriate. For instance, $\frac{7}{3}$ is an improper fraction since the numerator 7 is bigger than the denominator 3.
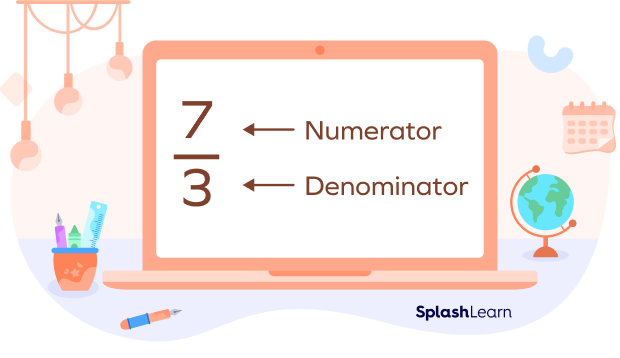
The improper fraction $\frac{7}{3}$ is displayed below. Note that $\frac{7}{3}$ in the mixed number form is $2\frac{1}{3}$. Thus, you can see that there are 2 wholes and fraction $\frac{1}{3}$.

Recommended Games
How to Convert a Mixed Number to an Improper Fraction
Let’s understand steps to convert a mixed number to an improper fraction with an example.
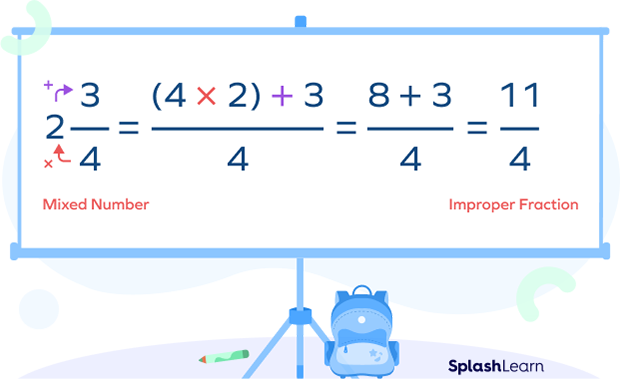
Example: Convert the mixed number $3\frac{4}{5}$ to improper fraction.
Step 1: Note down the whole number, numerator, and denominator in the given mixed number.
Here, the whole number $= 3$, Numerator $= 4$, Denominator $= 5$
Step 2: Multiply the denominator with the whole number.
Here, multiply 5 and 3.
$5 \times 3 = 15$
Step 3: Add the numerator to this product.
Add 15 and 4 (numerator): $15 + 4 = 19$
Step 4: This sum represents the numerator of the improper fraction. The denominator stays the same as the original fraction in the given mixed number.
Hence, $3\frac{4}{5} = \frac{19}{5}$.
Recommended Worksheets
How Do You Convert an Improper Fraction into a Mixed Fraction
Let’s understand steps to convert an improper fraction to a mixed number with an example.
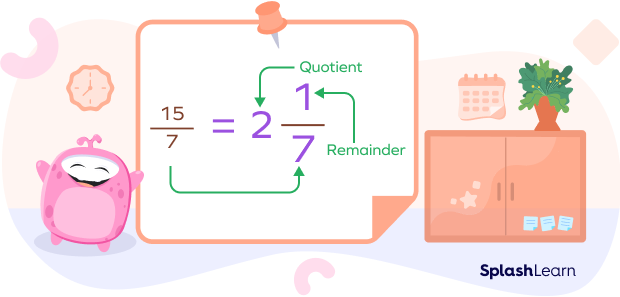
Example: Convert the improper fraction $\frac{15}{7}$ to a mixed number.
Step 1: Divide the fraction’s numerator by its denominator. Note down the quotient and the remainder.
Here, we divide 15 by 7.
We know that $(7 \times 2) + 1 =15$
Quotient $= 2$ and Remainder$ = 1$
Step 2: The quotient represents the whole number part of the mixed number. The remainder represents the numerator. The denominator stays the same.
Thus, $\frac{15}{7} = 2\frac{1}{7}$
Example 2: Consider the improper fraction $\frac{7}{3}$.
Mixed Number to Improper Fraction Conversion Chart
Let’s take a look at a few examples of mixed number to improper fraction conversion.
| Mixed Number | Improper Fraction |
|---|---|
| $1\frac{1}{2}$ | $\frac{3}{2}$ |
| $2\frac{3}{4}$ | $\frac{11}{4}$ |
| $3\frac{5}{3}$ | $\frac{14}{3}$ |
| $7\frac{1}{2}$ | $\frac{15}{2}$ |
| $4\frac{6}{7}$ | $\frac{34}{7}$ |
Adding Mixed Fractions
We can add mixed fractions easily by simply converting them into improper fractions.
Example: Find the sum: $4\frac{1}{3} + 5\frac{4}{3}$.
Convert both the mixed numbers to improper fractions.
$4\frac{1}{3} = \frac{(4 \times 3) + 1}{3} = \frac{13}{3}$
$5\frac{4}{3} = \frac{(5 \times 3) + 4}{3} = \frac{19}{3}$
Add the improper fractions by adding the numerators and keeping the same denominator.
$\frac{13}{3} + \frac{19}{3} = \frac{32}{3}$
Here, we can convert the improper fraction $\frac{32}{3}$ to a mixed number.
$\frac{32}{3} = 10\frac{2}{3}$
Thus, $4\frac{1}{3} + 5\frac{4}{3} = 10\frac{2}{3}$
When dealing with unlike fractions, we first find the Least Common Multiple (LCM) of the denominators and convert them to like fractions. They can then be added in the normal manner.
Example: $1\frac{1}{2} + 2\frac{1}{3}$
$1\frac{1}{2} = \frac{(2 \times 1) + 1}{2} = \frac{3}{2}$
$2\frac{1}{3} = \frac{(3 \times 2) + 1}{3} = \frac{7}{3}$
Here, LCM $(2,3)\; = 6$
$1\frac{1}{2} + 2\frac{1}{3} = \frac{3}{2} + \frac{7}{3}$
$= \frac{9}{6} + \frac{14}{6}$
$= \frac{23}{6}$
$= 3\frac{5}{6}$
Subtracting Mixed Fractions
We can subtract mixed fractions by converting them into improper fractions. The steps are similar to steps of adding mixed fractions; only the operation is different.
Conclusion
In this article, we learned how to convert a mixed fraction to an improper fraction and vice versa. Let’s solve a few examples to understand the concept better.
Solved Examples on Mixed Number to Improper Fraction Conversion
- Convert the mixed number $16\frac{2}{3}$ to an improper fraction.
Solution:
Multiply the denominator and the whole number.
$3 \times 16 = 48$
Add the remainder to it.
$48 + 2 = 50$
Numerator $= 50$
Denominator $= 3$
Thus, $16\frac{2}{3} = \frac{(16 \times 3) + 2}{3} = \frac{50}{3}$
- Convert the improper fraction $\frac{17}{5}$ to a mixed number.
Solution: Given improper fraction $= \frac{17}{5}$
First, divide 17 by 5.
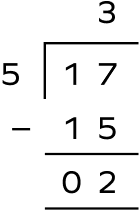
Quotient $= 3$ and Remainder $= 2$.
Thus, $\frac{17}{5} = 3\frac{2}{5}$
3. Convert the mixed number $1\frac{3}{7}$ to improper fraction.
Solution:
$1\frac{3}{7} = \frac{(1 \times 7) + 3}{7}$
$= \frac{7 + 3}{7}$
$= \frac{10}{7}$
Improper fraction $= \frac{10}{7}$
Practice Problems on Mixed Number to Improper Fraction Conversion
Mixed Numbers to Improper Fractions Conversion: Definition with Examples
Convert $5\frac{2}{3}$ to an improper fraction.
$5\frac{2}{3} = \frac{(5 \times 3) + 2}{3} = \frac{17}{3}$
The mixed number $6\frac{4}{7}$ is equivalent to which improper fraction?
$6\frac{4}{7} = \frac{(7 \times 6) + 4}{7} = \frac{46}{7}$
Therefore, $6\frac{4}{7} = \frac{46}{7}$
The mixed number is composed of a whole number and _____________.
The mixed number is composed of a whole number and a proper fraction.
Frequently Asked Questions on Mixed Number to Improper Fraction Conversion
What is a mixed fraction (mixed number)?
A mixed fraction is one that is represented by a whole number and a proper fraction. For example, $2\frac{1}{3}$ is a mixed number, where 2 is the whole number and $\frac{1}{3}$ is the proper fraction.
How are mixed fractions subtracted?
Mixed fractions can be subtracted using the same strategy as addition. In order to subtract mixed numbers, we must first transform them into improper fractions.
How can mixed fractions be added?
We can convert mixed fractions into improper fractions to add them.
The next step is to determine whether or not the denominators of the provided fractions are equal. If the denominators are not equal, we make them equal using the LCM method. Example: $2\frac{1}{3} + 2\frac{1}{5} = \frac{7}{3} + \frac{11}{5} = \frac{35 + 33}{15} = \frac{68}{15} = 4\frac{8}{15}$




































