What Is a Constant in Math?
A “constant” simply means a fixed value or a value that does not change. A constant has a known value.
If you measure the height of a wall or bookshelf at home, it will be a constant number. It won’t change. However, if you measure the height of a plant in a pot, it will keep changing as it grows. It’s not constant.
Take a look at the following sentences to understand this.
- There are 7 days in a week. Here,$\Rightarrow 7$ is a constant.
- July 4 is celebrated as Independence Day in the USA$\Rightarrow $. Here, 4 is a constant.
Constant: Definition
Constant is an entity whose value does not change throughout the calculation. It can be a number, decimal, or a fraction. A constant in math is often represented by a letter or a symbol or a number.
Examples of constants: 2, 1.5, $\sqrt{2}, \frac{3}{4}$
What Is a Constant Term in Algebraic Expression?
Constant term plays an important role In algebraic expressions or equations.
Consider an algebraic equation: $5x + 4y = 12$
Here,
- x, y are variables. Variable is a quantity that can be changed.
- 5 is the coefficient of x. 4 is the coefficient of y.
- 12 and 1 are constants.
5 and 4 are also numbers. Are they constants in this equation? — The answer is no. They are not constants. They are coefficients of the variables. We multiply the coefficient with whatever value gets assigned to the variable.
How to Recognize a Constant in Algebraic Expression
- It is a known value.
- It’s a stand-alone number.
- Even if the value is unknown, it is a set number.
- It involves fractions, decimals, whole numbers, all real numbers.
- An exponent is not a constant. For example, in the term $2^5$, 5 is not a constant.
Constant Numbers
All numbers are constant numbers. In math, real numbers, natural numbers, whole numbers, and integers are all constant numbers. They cannot take a different value.
Example: Ron read 5 pages of a book on Monday and 8 pages on Tuesday. How many pages did he read in two days?
Here, to find the total number of pages read by Ron, we have to add 5 and 8, which are constant numbers. They cannot take any other value.
Total number of pages read in the two days $= 5 + 8 = 13$ pages
Again, 13 is also a constant!
Arbitrary Constants
They are symbols that can have different values assigned to it, but are unaffected by changes in the values of a variable in the equation. Sometimes, English alphabets are used to represent fixed values, which are unknown.
Example: $y = mx + c$ is the general equation of a straight line, where m and c are arbitrary constants.
m: the slope of the line
c: the y intercept.
If $m = 2$ and $c = 1$, we get the equation of a line as $y = 2x + 1$.
Constant v. Variable
| Constant | Variable |
|---|---|
| It has a known value. | It does not have a fixed value. |
| The value is fixed and does not change over time. | It can take any value. |
| The symbols are usually numbers. Some famous constants such as pi are often identified using a standard symbol. | Different English alphabets are used to represent a variable. |
| Examples: $90,\; 6\;, \;-45\;$, etc | Examples: x, 5y, 9z |
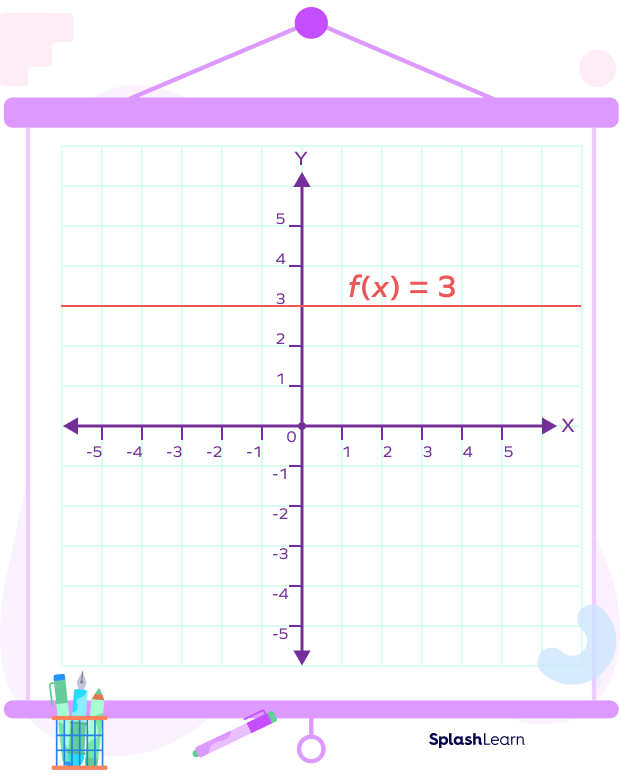
Constant Function
A constant function is a function that maps every number to a constant. Thus, the output is the constant number or the same for any input value.
We denote the constant function as: $f(x) = c$.
Suppose $c=3$
$f(x) = 3$
This is a constant function because the variable is x, and the constant 10 is not dependent on x. No matter what value of x we put in the equation, the output of the function will always be 10, since x does not appear in the function.
Mathematical Constants
We use the term mathematical constant to describe fixed, well-defined numbers. Some of mathematical constants include:
- $\pi = 3.1415926536$…
- $i^2 = \;-1$
- e = 2.7182818284…
- Pythagoras’ constant: $\sqrt{2} = 1.4142135623$…
- Theodorus’ constant: $\sqrt{3} = 1.7320508075$…
- Golden ratio: $\theta = 1.618033$…
Constants Written as Variables
A constant is commonly denoted a “c” to represent a fixed value when the exact value is not known in an expression or a word problem.
Here, “c” is the variable, but its value will always be a “fixed number” when actually writing a polynomial or expression.
Consider the quadratic equation of the form: $ax^2 + bx + c = 0$.
Here, a and b are coefficients of the variable x.
Here, c is a constant (the constant term of the polynomial).
Note: a, b, and c are also called parameters since they are used to represent a model or a family of quadratic equations. Parameters may take multiple values, but they do not change once assigned forming a particular function. When parameters are changed, we get a different function or equation.
Solved Examples on Constants
1. Find the constant in the algebraic expression $3x^2y\;-\;4xy + 5y +10$.
Solution:
Given expression: $3x^2y\;-\;4xy + 5y + 10$
The only term that is fixed is 10.
So, the constant is 10.
2. Why is 15 a constant?
Solution:
15 is a constant because 15 is an integer, it is a fixed value and it will not change.
3. In the equation $3x\;-\;5 = y$, which is the constant?
Solution:
There are three terms: $3x,\;-\;5$, and y.
x and y are variables.
$– 5$ is a fixed value or does not change.
So, $– 5$ is a constant.
5. James took 4 hours to travel from Place A to Place B, whereas Jack took 3 hours to cover the same distance. Which are the constants and variables in this statistical scenario?
Solution:
The distance between Place A and Place B is constant.
The time taken is a variable.
Practice Problems on Constants
What Is a Constant? Definition, Solved Examples, Facts
Which of the following is a constant?
20 is a constant as the value is fixed.
In the algebraic expression $4x\;-\;5 = 6y$, the constant is:
The value of $– 5$ is fixed. So, it is the constant.
Which of the following is not a constant?
The speed of a car always changes. It can never be a constant.
The constant term in the expression $8y^2\;-\;7y\;-\;9 = 14y$ is:
The constant term is $– 9$.
The constant term in the expression $6x + 7 = 10y$ is:
The constant term is 7.
Frequently Asked Questions on Constants
What is the difference between a coefficient and a constant?
Constant is the term in an expression that includes only numbers and whose value does not change. Coefficient of a variable can be defined as the number that is multiplied with the variable.
Can a constant be negative?
Yes. Negative numbers are real numbers. So, they can be constant.
What is a constant polynomial?
A constant polynomial is a polynomial with the highest degree 0.
Is 0 a constant?
Yes. 0 is a number. Its value does not change. Thus, 0 is a constant.
Can we write constants as coefficients in a polynomial?
A constant number can be written as a coefficient of a variable by writing the power of the variable as 0. Example: $6 = 6y^\circ$
















