What Are Alternate Angles?
When a transversal cuts a pair of parallel lines (or non-parallel lines), it forms different types of angles. Alternate interior angles are a set of non-adjacent angles on either side of the transversal.
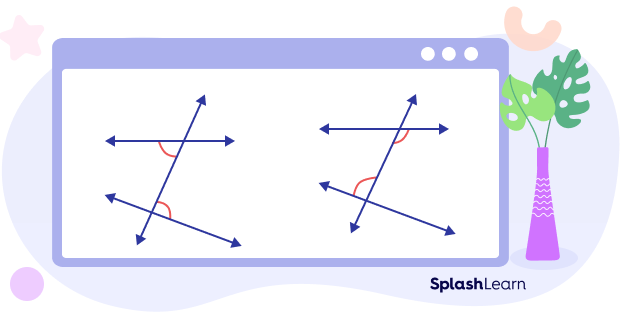
In each diagram given below, two parallel lines are cut by a transversal. All the angle pairs highlighted in the same color represent alternate angles. They are on alternate sides of the transversal. They don’t have common vertices.
Based on their position, they are further categorized as interior and exterior angles.
- Diagram on the left: Alternate interior angles
- Diagram on the right: Alternate exterior angles
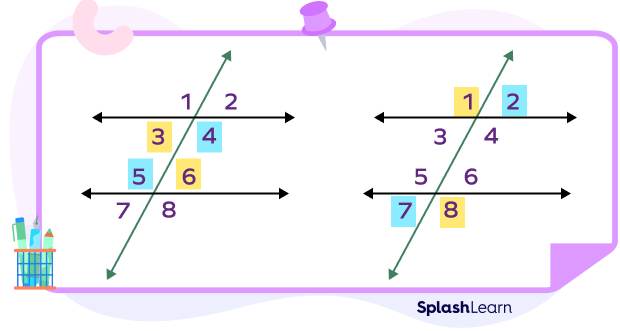
Observe that we can quickly spot a pair of alternate interior angles using the Z-shape. Take a look at the positions of alternate interior angles.
Thus, alternate interior angles are also sometimes known as Z-angles.
Recommended Games
Definition of Alternate Angles
Alternate angles are the non-adjacent angles that lie on the opposite sides of the transversal.
Recommended Worksheets
Types of Alternate Angles
There are two types of alternate angles based on their position with respect to the transversal and the parallel lines.
- Alternate Interior Angles
- Alternate Exterior Angles
Let’s understand the two types of alternate angles and their properties.
Alternate Interior Angles
The pair of angles that lie on the inner side (or the interior region) of the two parallel lines, but on the opposite sides of the transversal are known as alternate interior angles.
So, what do alternate interior angles look like? In the image given below, the angle-pairs highlighted in the same color represent the alternate interior angles.
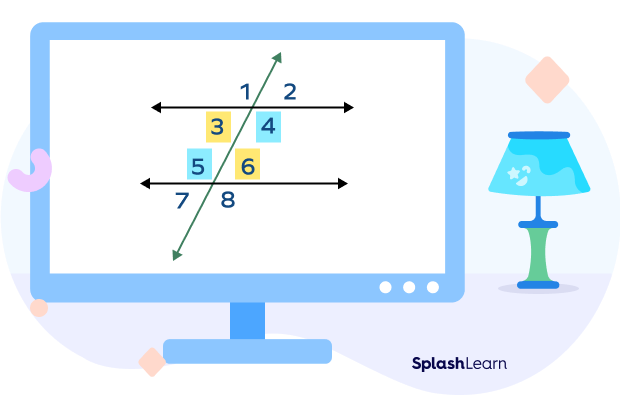
In the above image, the alternate interior angles are
$\angle 4$ and $\angle 5$
$\angle 3$ and $\angle 6$
Alternate Exterior Angles
The pair of angles that lie on the outside region of the two parallel lines, but on the opposite sides of the transversal are known as alternate exterior angles.
The angle-pairs highlighted in the same color represent the alternate exterior angles.
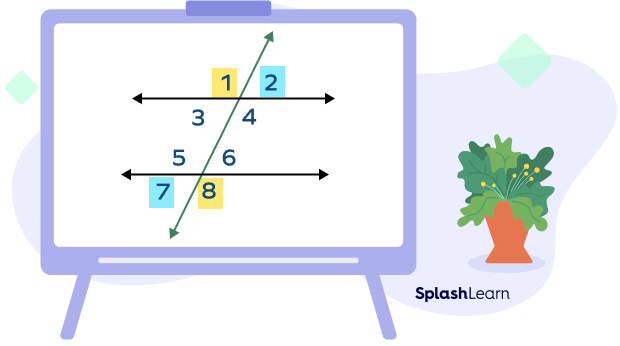
In the above image, the alternate exterior angles are
$\angle 1$ and $\angle 8$
$\angle 2$ and $\angle 7$
Alternate Interior Angles Theorem
Alternate Angles Theorem Statement: If two parallel lines are cut by a transversal, then the pairs of alternate interior angles formed are congruent.
Converse: If two lines are cut by a transversal such that the alternate interior angles are congruent, then the lines are parallel.
Alternate Exterior Angles Theorem
Alternate Exterior Angles Theorem Statement: If two parallel lines are cut by a transversal, then the pairs of alternate exterior angles formed are congruent.
Converse: If two lines are cut by a transversal so that the alternate exterior angles are congruent, then the lines are parallel.
Alternate Angles Theorem Proof
Assume that PQ and RS are the two parallel lines cut by a transversal LM.
a, b, c, d are the angles created by the transversal.
Since the corresponding angles are congruent, we have a corresponding pair for each of these angles, which are also labeled as a, b, c, and d.
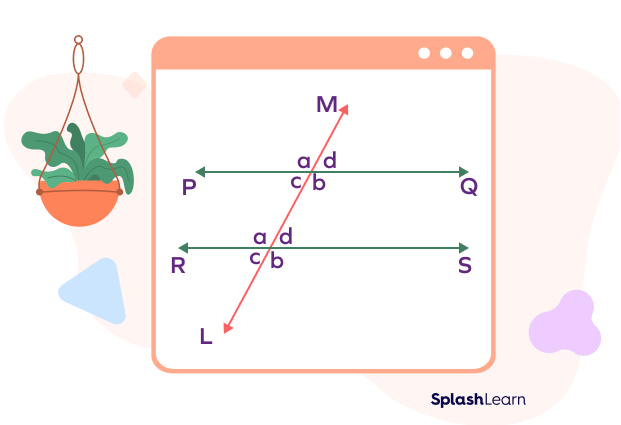
At the intersection point on the lines t and l,
$\angle a + \angle d = 180^{\circ}$ ( PQ is the straight line)— (1)
$\angle b + \angle d = 180^{\circ}$ ( LM is the straight line)— (2)
So, from (1) and (2), we get
$\angle a = \angle b$
Again, at the intersection point on the straight lines LM and RS,
$\angle a + \angle d = 180^{\circ}$ ( RS is the straight line)— (3)
$\angle a + \angle c = 180^{\circ}$ ( LM is the straight line)— (4)
So, from (3) and (4), we get
$\angle d = \angle c$
Therefore, it is concluded that the alternate interior angles are congruent.
Hence, proved.
Another way:
Here, we use the fact that the corresponding angles formed when a transversal cuts a pair of parallel lines are congruent.
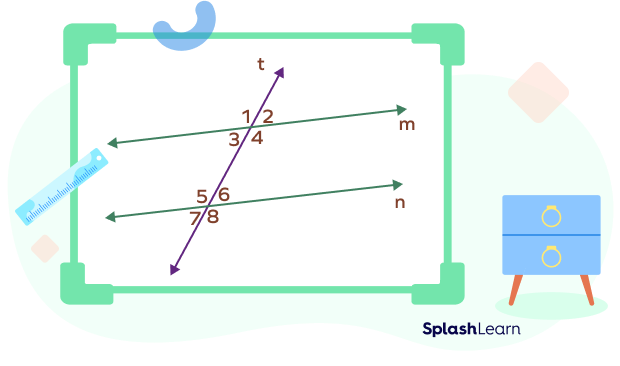
Here, $\angle 1 = \angle 5$ …corresponding angles
$\angle 1 = \angle 4$ …opposite angles
Thus, $\angle 4 = \angle 5$ …alternate interior angles
Also, $\angle 1 = \angle 4$ …opposite angles
$\angle 4 = \angle 8$ …corresponding angles
Thus, $\angle 1 = \angle 8$ …alternate exterior angles
Facts about Alternate Angles
- Alternate angles are angles that lie on opposite sides of the transversal line and have the same size.
- There are two different types of alternate angles, alternate interior angles and alternate exterior angles.
- The co-interior angles OR same-side interior angles add up to 180 degrees. The rule is sometimes remembered as “C angles” because the angles make a C shape.
- When two non-parallel lines intersect a transversal, the alternate interior angles formed will not be equal.
Conclusion
In this article, we learned about the alternate angles, types of alternate angles, and the theorems associated with it. Let’s solve some examples and practice problems based on each of these concepts.
Solved Examples on Alternate Angles
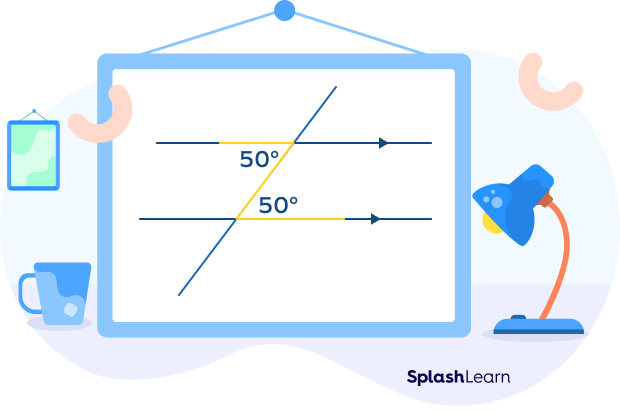
1. Use the alternate interior angles theorem to determine if the lines cut by the transversal are parallel.
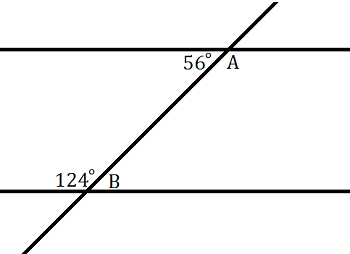
Solution:
Angle A and the angle measuring $60^{\circ}$ form a straight angle.
Thus, $m \angle A + 60^{\circ} = 180^{\circ}$
$m \angle A = 120^{\circ}$
Similarly, $\angle B$ and $120^{\circ}$ form a straight angle, so we know that
$m \angle B + 120 = 180$
$m \angle B = 60^{\circ}$
$\angle A$ and the original $120^{\circ}$ angle are alternate interior angles and are equal.
$\angle B$ and the original $60^{\circ}$ angle are also equal alternate interior angles.
So, going by the alternate interior angles theorem, the lines cut by the transversal must be parallel.
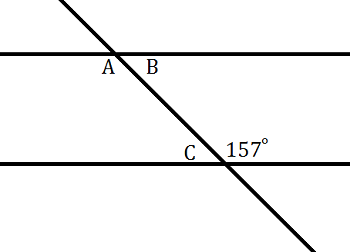
2. In the diagram given below, the lines cut by the transversal are parallel. Determine the measures of the angles A, B, and C.
Solution:
Angle A and 155 degrees are alternate interior angles, and so since the lines cut by the transversal are parallel, the measure of angle A is also 155 degrees.
Angle A and angle B form a straight angle, so $A + B = 180^{\circ}$.
Since $A = 155^{\circ},\; 155^{\circ} + B = 180^{\circ}$.
$B = 25^{\circ}$
Thus the measure of angle B is $25^{\circ}$.
Now, since angle B and angle C are alternate interior angles, we know that the measure of angle C is also $25^{\circ}$.
Thus, the measures of $\angle A,\; \angle B$ and $\angle C$ are $155^{\circ},\; 25^{\circ}$ and $25^{\circ}$ respectively.
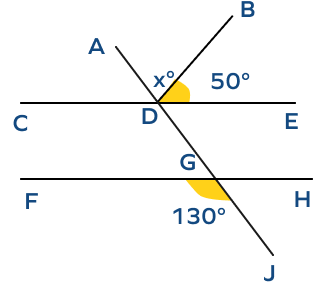
3. In the figure given below, CE is parallel to FH. Find the value of x.
Solution:
In the given figure, $\angle ADE$ and $\angle FGJ$ form a pair of alternate exterior angles.
By using alternate exterior angle theorem, we have, $\angle ADE = \angle FGJ$
So, $x^{\circ} + 50^{\circ} = 130^{\circ}$
$x = 130^{\circ} \;-\; 50^{\circ}$
$x = 80^{\circ}$
Therefore, the value of x is $80^{\circ}$.
4. Calculate the value for x and find the value of each angle.
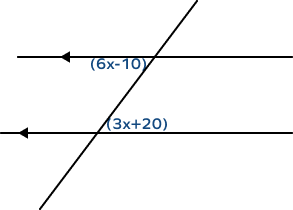
Solution:
Angles measuring $(6x \;-\; 10)$ and $(3x + 20)$ are alternate angles.
We know that alternate angles are equal.
So, $6x \;-\; 10 = 3x + 20$
$6x \;-\; 3x = 20 + 10$
$3x = 30$
$x = 10$
Substituting values in the equation of angles, we get,
$6x \;-\; 10 = 6 (10) \;-\; 10 = 60 \;-\; 10 = 50$
$3x + 20 = 3 (10) + 20 = 30 + 20 = 50$
Thus, the value of each labeled angle is $50^{\circ}$.
Practice Problems on Alternate Angles
Alternate Angles: Definition, Types, Theorem, Examples, Facts
From the image given below, identify the pair of alternate interior angles.
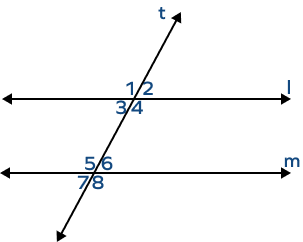
The pair of angles on the inner side of the two parallel lines but on the other side of the transversal are known as alternate interior angles. Thus, 3 and 6 are alternate interior angles.
In the given diagram, angles X and Y are
Angles X and Y are corresponding angles. They are formed at matching/corresponding corners with respect to the transversal.
Name the angle relationship in the diagram given below:
The pair of angles on the outside of the two parallel lines but on the other side of the transversal are known as alternate exterior angles. Thus, the angles 1 and 2 are alternate exterior angles.
Angles inside a pair of parallel lines that lie on the opposite sides of a transversal and are congruent are called _____.
The pair of angles on the inner side of the two lines but on the other side of the transversal are known as alternate interior angles.
Frequently Asked Questions about Alternate Angles
What are the corresponding angles?
When two lines are crossed by a transversal, the angles formed in the matching corners are called corresponding angles.When the two lines are parallel, corresponding angles are equal.
Take a look at the image given below:
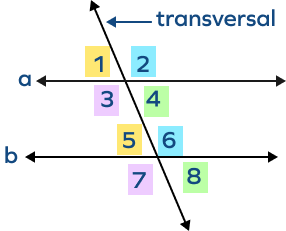
In the above image,
angle 3 corresponds to angle 7,
angle 4 corresponds to angle 8,
angle 1 corresponds to angle 5,
angle 2 corresponds to angle 6.
What is the difference between alternate angles and corresponding angles?
Alternate angles are defined as angles in a plane figure that lie on opposite sides of a transversal line and have the same measurements. In the image given below, 1 and 7, 4 and 6, 2 and 8, 3 and 5 are alternate angles.
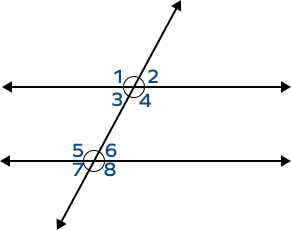
On the other hand, corresponding angles are angles that are in the same relative positions along the transversal line and still have the same measurements.
In the image given above, 1 and 5, 4 and 8, 2 and 6, 3 and 7 are pairs of corresponding angles.
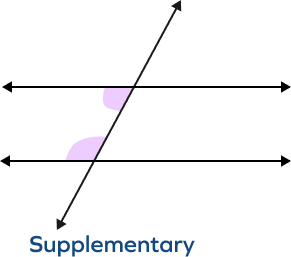
What are the same side interior angles?
The same side interior angles formed by a transversal cutting two parallel lines are shown in the figure below. They are supplementary.
How do you prove that the sum of the three interior angles of a triangle is 180 degrees?
If we have a triangle, we can always draw two parallel lines as shown in the figure.
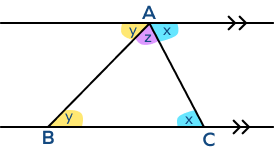
Now, AB and AC are the transversals. We know that alternate angles are equal. Therefore, the two angles labeled as x are equal. Also, the two angles labeled y are equal.
We know that x, y, and z together add up to 180 degrees, because together, these are just angles in a linear pair or the angles around the straight line. So, $x + y + z = 180^{\circ}$





























