Superset in Maths: Definition, Symbol, Properties, Example

What Is a Superset?

We call set B a superset of set A if B contains all the elements of A. In other words, if A is a subset of B, then B is the superset of A.

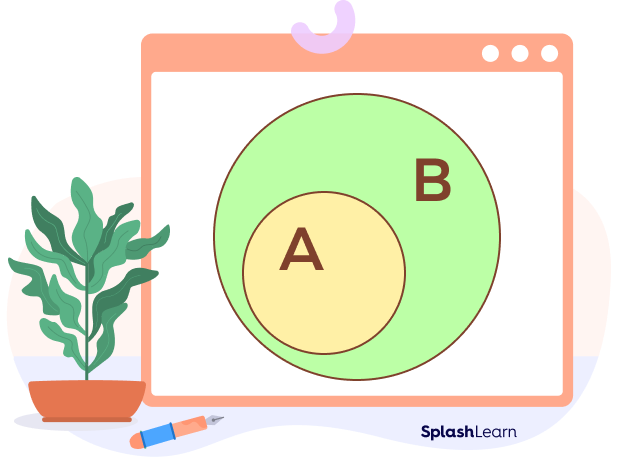
We know that set A is called a subset of set B if all the elements of A are present in B. Then what does superset mean? Let’s understand this with a Venn diagram.

In the following figure, set B contains set A. 

A is a subset of B. 

B is a superset of A.

Superset Meaning

In mathematics, a superset is a set that consists entirely of the elements of the smaller set. If P is the superset of Q, we can infer that Q is the smaller set and P contains all elements of Q.

Superset Definition

A superset of a given set is a set that contains all the elements of the given set.

If B is a superset of A, then A is a subset of B.

Example: A = {1, 2}, B= {1, 2, 3, 4, 5}

B contains all the elements of A.

Thus, B is a superset of A.

Also, A is a subset of B.

What Is a Proper Superset?

The proper superset or a strict superset is a superset that contains all the elements of the smaller set but also has some extra elements of its own. 

If set B is the proper superset of set A, then all the elements of set A are in B, but set B must contain at least one element that is not present in set A.

Suppose we have two sets: 

A={1, 3, 5}

B={1, 3, 4, 5}

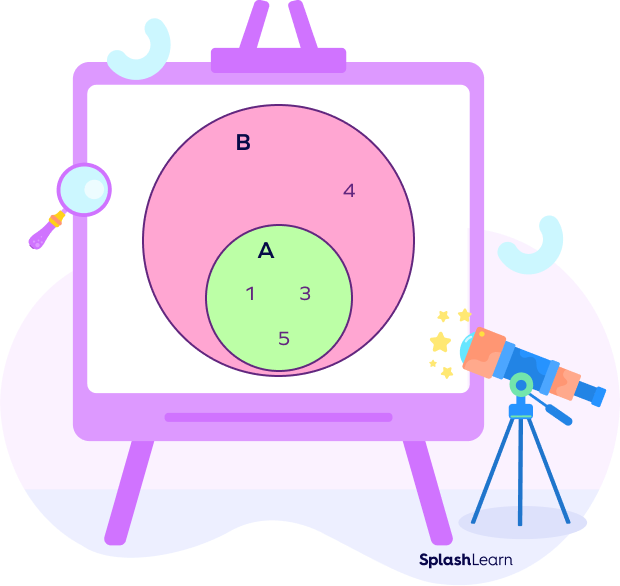
Here, B is a superset of A.

We can see that B is not exactly equal to A. 

B contains the element 4, which is not present in A.

Such a superset is called a proper superset. 

Superset Symbol

The symbols “⊃” or “⊇” are used to denote the superset. 

• B ⊃ A means that B is a proper superset or a strict superset of set A such that A B (the sets are not equal.)

• B ⊇ A means that B is a superset of A, but the possibility of A = B is also considered. 

Properties of Superset

• Empty set contains no elements. So, every set is a superset of the empty set.

For any set A, we have  A ⊃ ∅. 

• Every set is a superset of itself.

For any set A, we have A ⊇ A.

• For the given two sets (P and Q), if P ⊂ Q, then P ⊃ Q is true, indicating that subset and superset are opposite to one another.
• If B is a superset of A, then

A ∩ B = A

A ∪ B = B

• The cardinality of the superset is always greater than or equal to the other set. 

Difference between Superset and Subset

Subset	Superset
If A is a subset of B, the set A is contained in B.	If B is a superset of A, then B contains A.
A ⊂ B means that A is a proper subset of A.	B ⊃ A means that B is a proper or strict superset of A.
Subset is a smaller set compared to the other set.	Superset is a bigger set compared to the other set.
If A ⊂ B, then B ⊃ A.	If B ⊃ A, then A ⊂ B.
The empty set is a subset of every set.	Every set is a superset of the empty set.
Every set is a subset of itself.	Every set is a superset of itself.

Facts on Superset

Conclusion

A superset is a set that contains almost all of the components of a smaller set that it is composed of. We understand that if B is contained within A, then A includes B. The following solved examples and practice problems will further ease understanding of the concept of supersets. 

Solved Examples on Superset

1. Let Y= {1, 2, 3, 4, 5} and X= {1, 3, 5}. Identify the superset.

Solution:

Y= {1, 2, 3, 4, 5} and X={1, 3, 5}

Every element of X is also an element of Y. 

The set Y contains the set X.

Y ⊃ X

Hence, Y is the proper superset of X.

2. E is the set of positive even integers. Write two supersets of E.

Solution:

E is the set of positive even integers.

E = {2, 4, 6, 8, 10, …}

We know that the set of integers is given by

Z = {…, -3, -2, -1,0, 1, 2, 3, 4, …}

Thus, Z is a superset of E.

The set of natural numbers is given by

N = {1, 2, 3, 4, 5, …}

Thus, N is a superset of E.

3. Let P is a set of all polygons. Identify the sets for which P will be a superset. 

i) Set of convex polygons

ii) Set of quadrilaterals

iv) Set of triangles 

v) Set of circles

vi) Set of 2D shapes

Solution: 

P is a set of all polygons. 

In geometry, a polygon can be defined as a flat or plane, two-dimensional closed shape bounded with straight sides. It does not have curved sides. Also, we need at least three sides to form a polygon.

i) Set of convex polygons will be a subset of the set P. Thus, P is a superset of the set of convex polygons.

ii) All quadrilaterals are polygons. So, the set P is a superset of the set of quadrilaterals.

iii) Triangles are the polygons with three sides. So, P is a superset of the set of triangles.

iv) Circles are not polygons since they are not formed by straight lines. Thus, P is not a superset of the set of circles.

v) Polygons are 2D shapes, but 2D shapes contain polygons and many other shapes like circles. So, the set of polygons is a subset of the set of 2D shapes. The set of 2D shapes is a superset of the set of polygons.

4. If A = {4, 5, 7, 8} and B = {4, 7, 8}, justify why A is a proper superset of B. 

Solution:

A = {4, 5, 7, 8}

B = {4, 7, 8}

A is the proper superset of B because all elements of B are in A. The set A also has one extra element, 5, which is absent in B. 

Practice Problems on Superset

Frequently Asked Questions about Superset