What Is the Midpoint of a Line Segment?
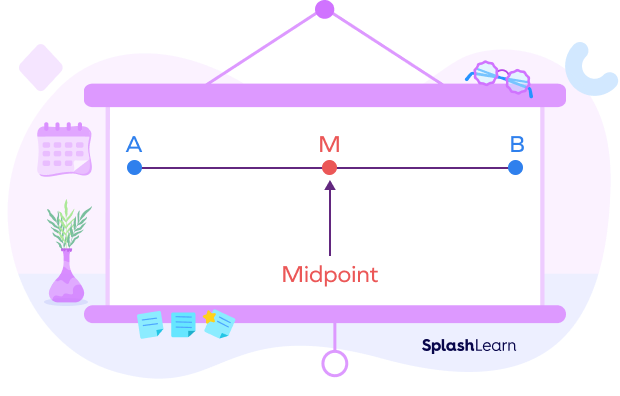
The midpoint of a line segment is the point that lies exactly at the center of a line segment, dividing it into two equal parts. The bisector of a line segment passes through the midpoint.
In the diagram shown below, the point M is the midpoint of line segment AB.
The points A and B are endpoints of AB.
What Is the Midpoint Formula?
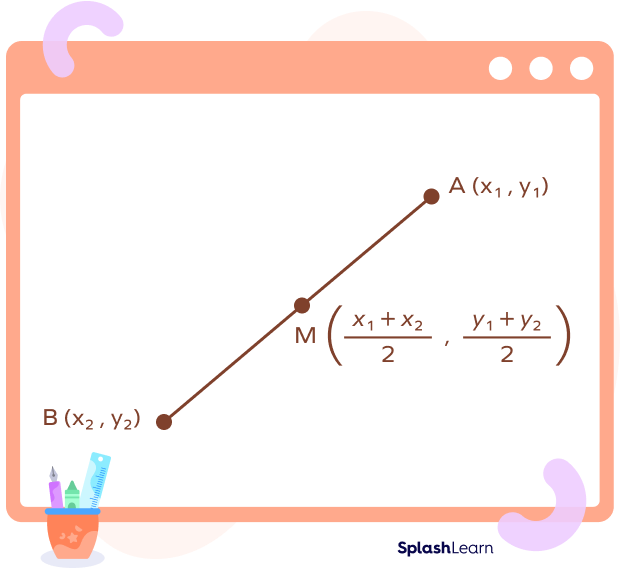
The midpoint formula is used to find the coordinates of the midpoint of a line segment using the coordinates of the endpoints.
Suppose a line segment has two endpoints, P(x1, y1) and Q(x2, y2). The midpoint formula defines the coordinates of the midpoint M(x3, y3) as
$x_{3} = \frac{x_{1} + x_{2}}{2}$
$y_{3} = \frac{y_{1} + y_{2}}{2}$
Thus, the coordinates of the midpoint M are $(\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$.
How to Find the Midpoint
Let’s discuss methods that are commonly used to determine the midpoint of a line segment.
1. Formula Method
Use the midpoint formula to find the coordinates of the midpoint.
Example: The points P(-4, 2) and Q(2, 2) are the endpoints of the line segment PQ.
So, to find the midpoint of the line segment, we use the formula:
$(x, y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$(x, y) = (\frac{-4 + 2}{2},\; \frac{2 + 2}{2})$
$(x, y) = (-1, 2)$
Thus, M(-1, 2) is the midpoint of the line segment PQ.
2. Graph Method
Using the graph, find the length of the line segment. Divide the length of the segment by 2. Measure this distance from any one of the endpoints to find the midpoint.
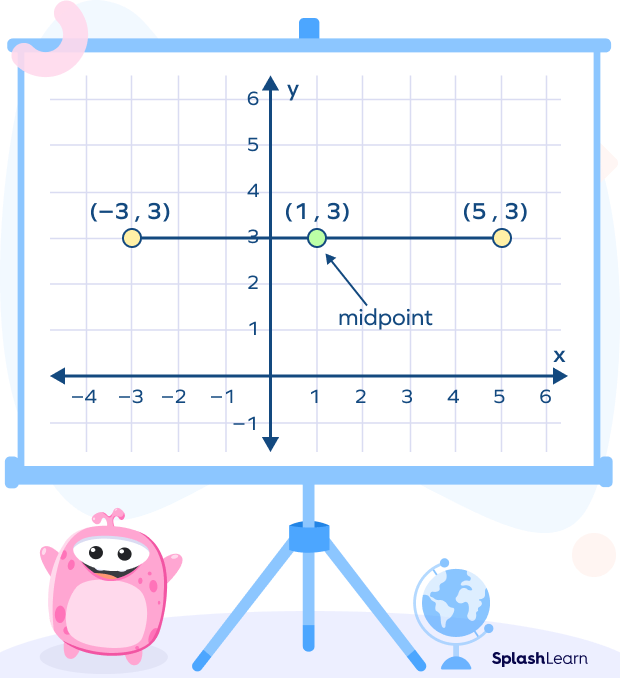
Example: Consider the line segment with endpoints (-3, 3) and (5, 3).
Length of the line segment = 8 units
$8 \div 2 = 4$
If you count 4 units to the right from (-3, 3), you land on (1, 3).
(-3 + 4, 3) = (1, 3)
If you count 4 units to the left from (5, 3), you land on (1, 3).
(5 – 4, 3) = (1, 3)
Therefore, the point (1, 3) is the midpoint.
Other Important Formulas related to the Midpoint
There are a number of formulas related to the midpoint formula. Some of them are mentioned below:
- Distance formula:
To calculate the distance between two points with coordinates (x1, y1) and (x2, y2), use the following formula:
$d = \sqrt{(x_{2} \;-\; x_{1})^{2} + (y_{2} \;-\; y_{1})2}$
- Slope formula:
To calculate the slope of a straight line passing through two points (x1, y1) and (x2, y2), use the following formula:
$m = \frac{y_{2}\; -\; y_{1}}{x_{2} \;-\; x_{1}}$
- Centroid of Triangle Formula:
The point of intersection of the medians of a triangle is the centroid of a triangle. The vertices of a triangle (x, y) are given as:
$x = \frac{(x_{1} + x_{2} + x_{3})}{3}$
$y = \frac{(y_{1} + y_{2} + y_{3})}{3}$
- Equation of a Line:
To find the equation of a line passing through two points (x1, y1) and (x2, y2), you can use the slope formula and point-slope form of the equation:
$y\;-\;y_{1} = m(x\;-\;x_{1})$
- Section Formula:
- Internal section formula: It is used to find the coordinates of a point R (x, y) dividing a line segment joining points A(x1, y1) and B(x2, y2) internally in the ratio m : n.
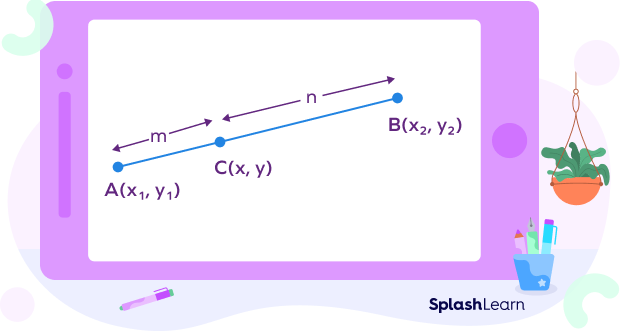
$x = \frac{(mx_{2} + nx_{1})}{(m + n)}$
$y = \frac{(my_{2} + ny_{1})}{(m + n)}$
- External section formula: It is used to find the coordinates of a point R (x, y) dividing a line segment joining points A(x1, y1) and B(x2, y2) externally in the ratio m : n.
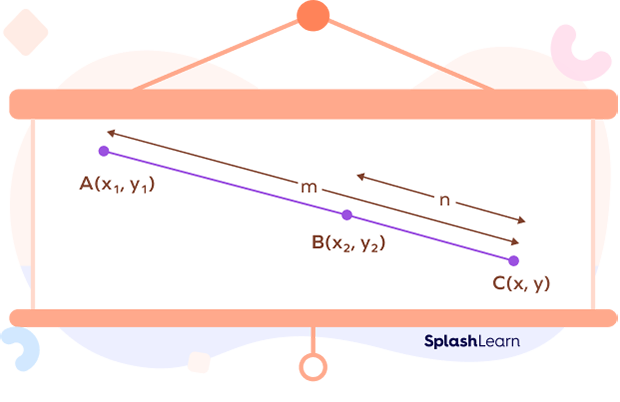
$x = \frac{mx{2} \;-\; nx_{1}}{m \;-\; n}$
$y = \frac{my_{2} \;-\; ny_{1}}{m \;-\; n}$
Midpoint Formula Derivation
Consider the line segment AB with endpoints A(xA, yA) and B(xB, yB).
Let’s write the coordinates of the midpoint M as M(xM, yM).
Consider the points on the X-axis and Y-axis given by
XA= (xA,0), YA= (0,yA)
XB= (xB,0), YB= (0,yB)
XM= (xM,0), YB= (0,yM)
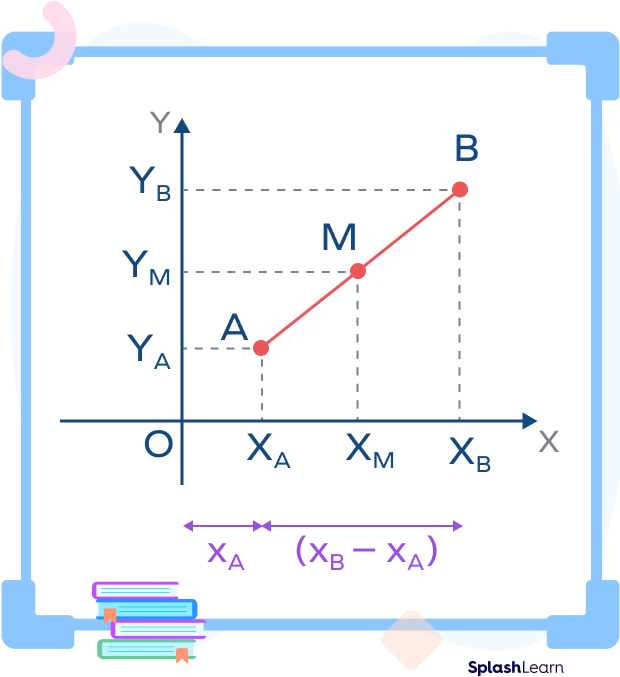
Distance OXM = X-coordinate of midpoint M = xM
xM = OXA + XAXM
xM $= x_{A} + \frac{x_{B}\;-\;x_{A}}{2}$
xM $= \frac{2x_{A}}{2} + \frac{x_{B}\;-\;x_{A}}{2}$
xM $= \frac{x_{B} + x_{B}}{2}$
Similarly,
Distance OYM = Y-coordinate of midpoint M = yM
yM = OYA + YAYM
yM $= y_{A} + \frac{y_{B}\;-\;y_{A}}{2}$
yM $= \frac{2yA}{2} + \frac{yB-yA}{2}$
yM $= \frac{y_{A} + y_{B}}{2}$
Facts about Midpoint Formula
- The point of intersection of the medians of a triangle is called the “centroid of the triangle.” Its coordinates are given by
$(x, y, z) = (\frac{x_{1} + x_{2} + x_{3}}{3},\; \frac{y_{1} + y_{2} + y_{3}}{3})$ - The midpoint is considered the center of symmetry for its line segment.
- The midpoint divides a line segment in the equal ratio of 1:1.
Conclusion
In this article, we learned about the midpoint, midpoint formula and its significance in geometry for finding the coordinates of the midpoint. Understanding the midpoint formula has various mathematical applications. For hands-on practice and better understanding, let’s now explore a few examples and MCQs.
Solved Examples on the Midpoint Formula
Example 1: The midpoint of a line segment AB is (2, –1). Find the coordinates of point B if that of point A are (–3, 5).
Solution:
Let’s write the coordinates of point B as (x2, y2).
Putting it in the midpoint formula, we get
$(x, y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$\frac{(\;-\;3 + x_{2} )}{2} = 2$
Thus, $x_{2} = 7$
$\frac{(5 + y_{2})}{2} = \;-\;1$
Thus, $y_{2} = \;-\;7$
So, the coordinates of point B are (7, -7).
2. What are the coordinates of the midpoint of a line segment whose endpoints are (4, 1) and (–2, 3)?
Solution:
Let’s write the coordinates of the midpoint as (x, y).
We will use the endpoints of the line segment to find the midpoint.
$(x, y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$(x, y) = (\frac{4 + (-2)}{2},\; \frac{1 + 3}{2})$
(x, y) = (1, 2)
So, the coordinates of the midpoint are (1, 2).
3. If the midpoint of the line segment AB is (3, 4) and point A is (5, 6), what will be the coordinates of point B?
Solution:
Let’s write the coordinates of point B as (x, y).
Let us apply the given values in the midpoint formula:
$(x, y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$x = \frac{x_{1} + x_{2}}{2}$
$y = \frac{y_{1} + y_{2}}{2}$
Given: A = (5, 6) and midpoint = (3, 4)
$3 = \frac{5 + x_{2}}{2}$
$x_{2} = 1$
$4 = \frac{6 + y_{2}}{2}$
$y_{2} = 2$
So, the coordinates of point B are (1, 2).
4. Find the midpoint of the line segment that connects the endpoints (–3, 5) and (7, –2).
Solution:
Let’s write the coordinates of the midpoint M as M(x, y).
We can calculate the value of M(x, y) by applying the midpoint formula:
$x = \frac{\;-\;3 + 7}{2} = 2$
$y = \frac{5 \;-\; 2}{2} = 1.5$
So, the M(x, y) midpoint coordinates of the line segment are (2, 1.5).
5. If the midpoint of a line segment with endpoints (1, h) and (7, 5) is (4, –1), find the value of h.
Solution:
To find the value of h, we will use the formula for the y-coordinate of the midpoint.
$y = \frac{y_{1} + y_{2}}{2}$
Given: Midpoint = (4, -1)
Endpoints are (1, h) and (7, 5).
$y = \frac{y_{1} + y_{2}}{2}$
$\;-\;1 = \frac{h + 5}{2}$
$h = \;-\;7$
Therefore, the y-coordinate of the endpoint (1, h) is -7.
Practice Problems on the Midpoint Formula
What Is the Midpoint Formula? Examples, Derivation, Facts, FAQs
What is the midpoint of the line segment with endpoints (2, 4) and (6, 10)?
$(x_{1},\; y_{1}) = (2,\; 4)$ and $(x_{2},\; y_{2}) = (6,\; 10)$
$(x, y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$(x,\; y) = (\frac{2 + 6}{2},\; \frac{4 + 10}{2})$
$(x,\; y) = (4,\; 7)$
The midpoint divides the line segment in the ratio
The midpoint lies on the bisector of a line segment. It divides the line segment into an equal ratio of 1:1.
Which option shows the correct midpoint of the line segment joining the points (1, -2) and (-5, 4)?
We can calculate the midpoint coordinates by using the formula:
$(x,\; y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$(\frac{1 \;-\; 5}{2},\; \frac{-2 + 4}{2}) = (-2,\; 1)$
Therefore, (-2, 1) are the coordinates of the midpoint of the line segment joining the points (1, -2) and (-5, 4).
What is the midpoint of a line segment connecting (3, 5) and the origin?
The line segment joins the points (3, 5) and (0, 0).
$(x,\; y) = (\frac{x_{1} + x_{2}}{2},\; \frac{y_{1} + y_{2}}{2})$
$(x,\; y) = (\frac{3 + 0}{2},\; \frac{5 + 0}{2})$
$(x,\; y) = (1.5,\; 2.5)$
Frequently Asked Questions about Midpoint Formula
What are the uses of the midpoint formula?
The midpoint formula is mainly used to find the coordinates of the midpoint of a given line segment where the coordinates of the endpoints are known.
How can we determine the distance from the midpoint of a line segment to an endpoint?
The length of a line segment is divided in exactly half by its midpoint. So, we can easily determine the distance from the midpoint of a line segment to an endpoint by first calculating the midpoint.
Does the midpoint lie on the perpendicular bisector?
Yes, the midpoint of a line segment lies on the bisector and the perpendicular bisector of a line segment.
Is the midpoint of a line segment unique?
The midpoint of a line segment is unique.
What is the definition of midpoint in geometry?
The midpoint in geometry refers to the exact center or middle point of a line segment. It divides the segment into two equal halves, with each half having the same length.
















