What Is Associative Property in Math?
Associative property is defined as, when more than two numbers are added or multiplied, the result remains the same, irrespective of how they are grouped.
For instance,
2 × (7 × 6) = (2 × 7) × 6
2 + (7 + 6) = (2 + 7) + 6
Recommended Games
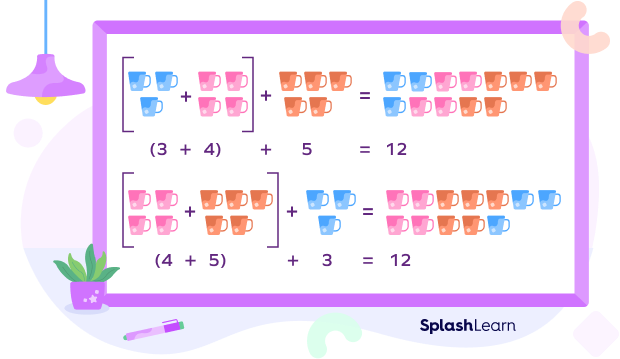
Associative Property of Addition
Associative property for addition implies that regardless of how numbers are grouped, the final sum of the numbers will remain the same. This can be expressed as:
(x + y) + z = x + (y + z)
Recommended Worksheets
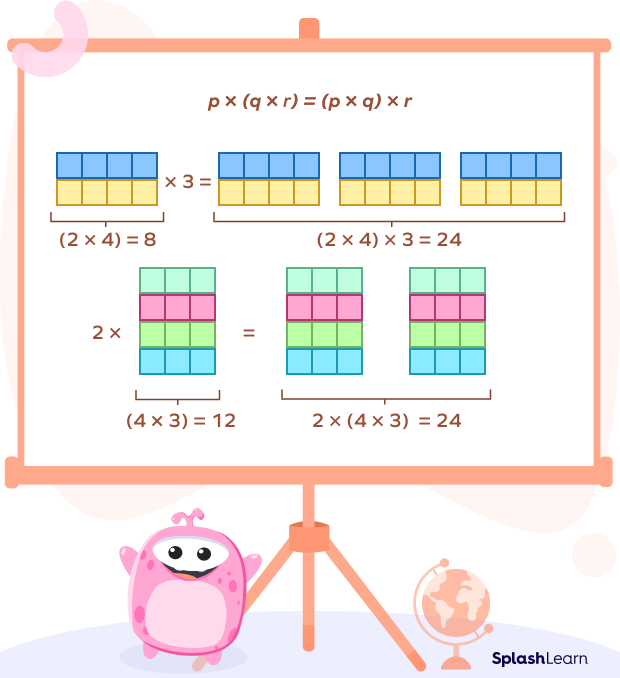
Associative Property of Multiplication
Associative property for multiplication implies that regardless of how numbers are grouped, the final product of the numbers will remain the same. This can be expressed as:
p × (q × r) = (p × q) × r
Difference between Associative Property and Commutative Property
Commutative Property
Commutative Property implies that when multiplication or addition is performed on two numbers, the result remains the same, irrespective of their arrangement.
Now, when you know about both the properties, you must have figured out that the only difference lies in the number of numbers involved in the operation.
Two numbers are involved in commutative property and more than two numbers are involved in associative property.
Is Associative Property Applicable to Division and Subtraction?
You must be wondering why these properties don’t apply to division and subtraction. Let’s take an example to answer this “Why?”.
In the case of subtraction:
12 – (6 – 2) = 12 – 4 = 8
(12 – 6) – 2 = 6 – 2 = 4
This implies, 12 – (6 – 2) ≠ (12 – 6) – 2. So, the associative property is not applicable for subtraction.
In the case of division:
(24 ÷ 4) ÷ 2 = 6 ÷ 2 = 3
24 ÷ (4 ÷ 2) = 24 ÷ 2 = 12
This implies, (24 ÷ 4) ÷ 2 ≠ 24 ÷ (4 ÷ 2). So, the associative property is not applicable for the division.
Fun Fact!
The associative property gets its name from the word “associate”, and it refers to the grouping of numbers.
Solved Examples On Associative Property
Example 1: If (30 × 20) × 15 = 9000, then use associative property to find (15 × 30) × 20.
Solution: According to the associative property of multiplication, (30 × 20) × 15 = (15 × 30) × 20.
Given that (30 × 20) × 15 = 9000,
(15 × 30) × 20 = 9000.
Example 2: Check whether the associative property of addition is implied in the following equations.
- 20 + (60 + 5) = (20 + 60) + 5
- 30 + (40 + 20) = (30 + 10) + 50
Solution:
- According to the associative property of addition, 20 + (60 + 5) = (20 + 60) + 5. Let’s check further.
LHS: 20 + (60 + 5)
20 +(65)
85
RHS: (20 + 60) + 5
(80) + 5
85
LHS = RHS. Thus, the associative property of addition is implied in this equation.
- According to the associative property of addition, 30 + (40 + 20) = (30 + 10) + 50. Let’s check further,
LHS: 30 + (40 + 20)
30 + (60)
90
RHS: (30 + 10) + 50
(40) + 50
90
LHS ≠ RHS. Thus, the associative property of addition is not implied in this equation.
Example 3: According to the associative property, fill in the missing number.
(5 + 10) + 4 = (5 + 4) + __ = 14
Solution: According to the associative property, when more than two numbers are added, the result remains the same irrespective of how they are grouped. Hence, (5 + 10) + 4 = (5 + 4) + 10 = 14
Practice Problems On Associative Property
What is Associative Property? Definition With Examples
Complete the following equation: 2 × ( _ × 6) = (2 × 5) × _
According to the associative property, when more than two numbers are multiplied the result remains the same, irrespective of how they are grouped.
Hence, $2 × (5 × 6) = (2 × 5) × 6$
Fill in the blanks: 20 + 9 + _ = 9 + 5 + _
According to the associative property, when more than two numbers are added, the result remains the same irrespective of how they are grouped.
Hence, $20 + 9 + 5 = 9 + 5 + 20$
Which of these equations is correct?
The associative property holds for addition and multiplication only. The property does not apply to subtraction or division, and hence the other equations are incorrect.
Frequently Asked Questions On Associative Property
Associative property of addition and multiplication can be used for how many numbers?
The associative property of addition and multiplication holds true for 3 or more numbers.
Does the associative property apply to fractional numbers?
Yes, the associative property of addition and multiplication holds true for fractional numbers.
Does the associative property apply to an equation that has 0 in it?
Yes, the associative property holds true for an equation that has 0 in it.




































