What is the Addition Property of Equality?
The addition property of equality is an important concept for solving algebraic equations. In algebra, an equation is a mathematical statement that shows that two mathematical expressions are equal. For instance, $5 \times 2 = 10$ is an equation, in which $5 \times 2$ and 10 are two expressions separated by an “equal $(=)$” sign. Think of an equation like a balanced weighing scale. The left side and the right side should be equal for it to be balanced.
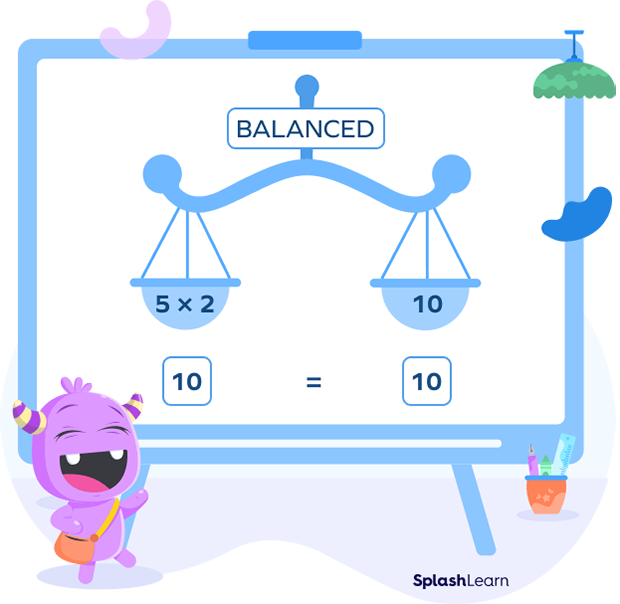
Now, to keep the scale balanced, if any mathematical operation is applied on one side of an equation, then it must be applied on the other side of an equation as well. This is called the property of equality.
The properties that do not impact the equality in an equation are called the properties of equality. There are many properties of equality but in this article, we will learn about “the addition property of equality.”
Addition Property of Equality Definition
The addition property of equality states that if the same number or value is added to both sides of an equation, then the equality still holds true after addition. Adding the same number on both sides of an equation does not affect the equality.
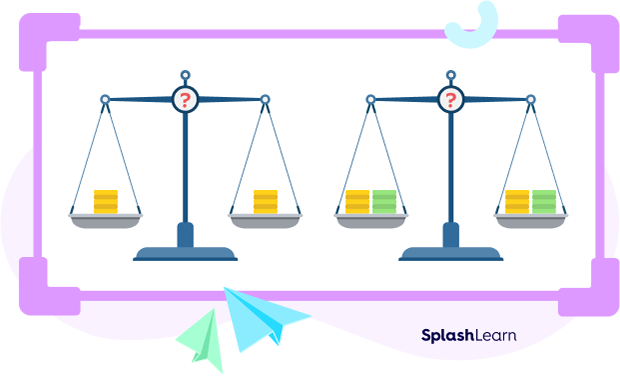
We can again consider the real-life example of the weighing scale. Suppose that initially there are 5 coins on both sides which balances the scale. (Observe the image on the left.)
If we add 5 more coins to one of the scales, then to keep the scales balanced, we must add 5 coins to the other scale as well. So, we will have 10 coins each on both sides of the balance. (Observe the image on the right.)
Recommended Games
Addition Property of Equality Formula
Consider an equation $x = y$, where x and y are real numbers.
If a real number “c” is added to x, the left-hand side (L.H.S.) of the equation, then we must add “c” to y, the right-hand side (R.H.S.) of the equation. So, the formula is given by,
If $x = y$ , then $x + c = y + c$
Thus, the addition property of equality states that the equality holds or the equation is balanced if the same number is added to both sides of the equation.
Recommended Worksheets
Verification of Addition Property of Equality
We know the addition property of equality, so we will verify it by taking a few examples.
Arithmetically, we know that $13 + 9 = 22$.
Now, if we add 7 to both sides of the equation, we have
L.H.S $= 13 + 9 + 7 = 29$
R.H.S $= 22 +7 = 29$
L.H.S $=$ R.H.S
It implies that when the same number is added to both sides of an equation, the equality still holds or the equation is balanced.
Addition Property of Equality for Fractions
The addition property of equality can also be applied to equations including fractions.
Consider an equation $\frac{a}{b} = \frac{p}{q}$, where $b,\; q \neq 0$ and $a,\; b,\; p,\; q$ are real numbers.
If we add the same fraction cd to both sides of the equation, the equation will stay balanced. Thus, we have
$\frac{a}{b} = \frac{p}{q}$
$\Rightarrow \frac{a}{b} + \frac{c}{d} = \frac{p}{q} + \frac{c}{d}$
The addition property in geometry (where side-lengths, angle measures, etc., are part of an equation) works the same way as it does in algebra.
Conclusion
In this article, we learned about the addition property of equality. The addition property of equality states that if we add the same number on both sides of an equation, then the equation remains balanced. Let’s solve a few examples of addition property of equality to understand the concept better!
Solved Examples for Addition Property of Equality
1. Let k be a real number such that $k\;-\;5 = 15$. Use the addition property of equality to find the value of k.
Solution: $k\;-\;5 = 15$
The addition property of equality states that we should add the same number to both sides of an equation.
To solve for k, we need to isolate the variable (keep only k on the left side and move the other terms on the right side). Add 5 to the left side of the equation.
$k\;-\;5 + 5 = 15 + 5$
$k = 20$
2. Martha has 7 marbles in each hand. Her best friend gave her 6 more marbles. Explain how she can balance the number of stickers in each hand.
Solution: Initially, Martha is holding 7 marbles in both hands.
$7 = 7$
She gets 6 more marbles. As 6 is an even number, she can divide the 6 marbles in two groups of 3. Now, she can hold 3 more marbles in each hand.
$7 + 3 = 7 + 3$
$10 = 10$
3. If x, y, and z are real numbers such that $y = z$ and $z = 7 \;-\; x$, then using the addition property of equality show that $x + y = 7$.
Solution: It is given that $y = z$ and $z = 7\;-\;x$
$z = 7\;-\;x$
Add x to both sides, we get
$z + x = 7\;-\;x + x$
$z + x = 7$
Since $y = z$
$y + x = 7$
4. Using the addition property of equality, find the value of x in the equation $2x \;-\; 3 = 6$.
Solution: The given equation is $2x \;-\; 3 = 6$
Add 3 to both sides, we get
$2x \;-\; 3 + 3 = 6 + 3$
$2x = 9$
Divide both sides by 2, we get
$x = \frac{9}{2}$
Practice Problems on Addition Property of Equality
Addition Property of Equality: Definition with Examples
There are two packets of flour of the same weight. If 15 ounces of flour is added to each bag, what will be the equation of the new weights of the packets?
Let the weight of the flour packets be a and b respectively. Since both packets have the same weight, so $a = b$.
On adding 15 ounces to each packet, we get $a + 15 = b + 15$.
If $p\;-\;8 = q\;-\;8$, then choose the correct option:
According to the addition property of equality, if $a = b$ then $a + c = b + c$.
In the equation $p\;-\;8 = q\;-\;8$, add 8 to both sides of the equation.
So, we get $p\;-\;8 + 8 = q\;-\;8 + 8$
$ \Rightarrow p = q$
For the equation, $a\;-\; \frac{2}{3} = \frac{3}{4}$, find the value of a.
On adding $\frac{2}{3}$ to both sides, we get
$a \;-\; \frac{2}{3} + \frac{2}{3} = \frac{3}{4} + \frac{2}{3}$
$a = \frac{17}{12}$
The addition property of equality is given by the formula:
The addition property equality states that we have to add the same number to both sides.
If $x = y$, then $x + c = y + c$.
Find the value of x for the algebraic equation $x \;-\; 22 = \;-27$.
On adding 22 to both the sides, we get
$x\;-\;22 + 22 = \;-27 + 22$
$x = \;-5$
Frequently Asked Questions on Addition Property of Equality
Can the number that we add to both sides be 0 in the addition property of equality?
Yes, the number that we add to both sides can be 0. Note that adding 0 to any number results in the number itself.
What is the difference between the addition property of equality and the subtraction property of equality?
The addition property of equality is the property that states that if a value is added to two equal quantities, then the sums are also equal, i.e., if $x = y$, then $x + c = y + c$.The subtraction property of equality is the property that states that if a value is subtracted from two equal quantities, then the differences are also equal i.e. if $x = y$, then $x\;-\;c = y\;-\;c$.
What is the addition property of inequality?
The addition property of inequality states that if the same number is added to both sides of any given inequality, then the inequality remains the same.
Can the number that we add to both sides be negative in the addition property of equality?
Yes, the number which we add to both sides can be negative.
What are the applications of the addition property of equality?
We can simplify, balance, and solve equations using the addition property of equality. It also helps in simplifying complex algebraic expressions.
How to solve the addition property of equality with fractions?
We can also apply the addition property of equality to fractions. Adding the same fractions on both sides of an equation maintains the equality.
Example: $x \;-\; \frac{1}{2} = \frac{3}{2}$
Thus, $x \;-\; \frac{1}{2} + \frac{1}{2} = 1 + \frac{1}{2}$
$x = \frac{3}{2}$




































