- What Is Additive Identity and Multiplicative Identity?
- Definition of Multiplicative Identity
- What Is the Difference between Additive and Multiplicative Identity?
- Solved Examples on Additive Identity vs Multiplicative Identity
- Practice Problems on Additive Identity vs Multiplicative Identity
- Frequently Asked Questions on Additive Identity vs Multiplicative Identity
What Is Additive Identity and Multiplicative Identity?
In mathematics, the identity property of addition states that when a number is added to zero, the sum will always be the number itself. The identity property of multiplication states that when a number is multiplied by 1, the product will be the number itself.
These two fundamental algebraic identities are called additive identity and multiplicative identity. The additive identity is 0 and the multiplicative identity is 1 for all the whole numbers, natural numbers, integers, & real numbers.
Recommended Games
What Is Additive Identity?
An additive identity is a number that, when added to any other number, gives the sum as the number itself. This property holds true for whole numbers, real numbers, integers, and rational numbers.
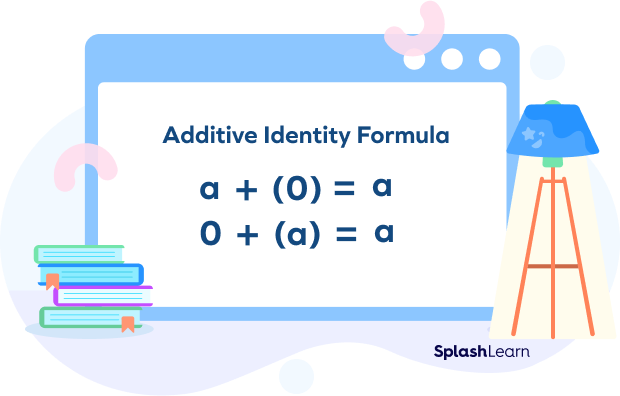
“Zero” is called the identity element for the real numbers under addition. The additive identity formula is expressed as
$a + 0 = a$
$0 + a = a$
where “a” can be any real number.
Recommended Worksheets
Definition of Additive Identity
An additive identity is defined as an element that, when added to a given element in a specified set, results in the number itself. 0 is the additive identity for the set of real numbers. The sum of any real number and 0 is the number itself.
Here are some examples of additive identity property:
- $3 + 0 = 3$
- $4.5 + 0 = 4.5$
- $-7 + 0 = -7$
From the above example of the property of addition, we get that adding 0 to any number gives us the number itself. This is called the Additive Identity Property of 0.
What Is Multiplicative Identity?
Multiplicative identity is a number whose product with any number is the number itself. Multiplicative Identity property states that any number multiplied by 1 results in the number itself. It is also called the identity property of multiplication. This is because the identity of the number remains the same.
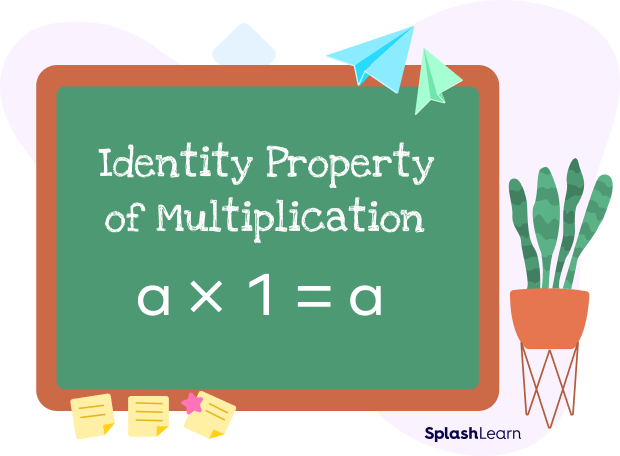
“1” is the multiplicative identity for the real numbers.
The multiplicative identity formula is expressed as
$a \times 1 = a$
where “a” can be any real number.
Definition of Multiplicative Identity
Multiplicative identity is an element that, when multiplied with another number, results in the number itself. The number 1 is known as the multiplicative identity for real numbers. Multiplying the number 1 with a real number always results in the number itself.
Here are some examples of the identity property of multiplication:
- $1 \times 3 = 3$
- $1 \times (\;-\;5) = (\;-\;5)$
- $1 2.3 = 2.3$
We get the same number as the result when we multiply it by 1. The number does not change, which means it keeps its identity, which is the reason for the name of the property. We say that 1 is the multiplicative identity.
What Is the Difference between Additive and Multiplicative Identity?
An additive identity is a number that when added to any number gives the sum as the number itself. The number 0 has the additive identity property. On the other hand, a multiplicative identity is a number that when multiplied to any number gives the product as the number itself. The number 1 has the multiplicative identity property.
| Additive Identity vs. Multiplicative Identity | |
| Additive Identity | Multiplicative Identity |
| An additive identity is a number which when added to any other number, gives the sum as the number itself. | A multiplicative identity is a number which when multiplied to any number, gives the product as the number itself. |
| For real numbers, the additive identity is 0. | For real numbers, the multiplicative identity is 1. |
| It is used in the addition operation. | It is used in the multiplication operation. |
| The additive identity formula for any real number ““a” is expressed as $a + 0 = a$ $0 + a = a$ | The multiplicative identity formula for any real number “a” is expressed as $a \times 1 = a$ |
Facts about Additive Identity vs Multiplicative Identity
The identity property states that when a number is combined with an identity, either 0 or 1, the end result will be the number itself.
Additive identity for any real number is zero, whereas the multiplicative identity for any real number is one.
Suppose a is any number other than a natural number, then we can express additive identity mathematically as: $a + 0 = a = 0 + a$
The multiplicative identity formula is expressed as $a \times 1 = a$. Here, “a” can be any real number.
Conclusion
In this article, we have studied additive and multiplicative identities and the difference between them. Let’s solve some examples and practice problems based on the same.
Solved Examples on Additive Identity vs Multiplicative Identity
1. If $a + 0 = 6$, then what is the value of a?
Solution:
Given: $a + 0 = 6$
By additive identity, if 0 is added to a number, it returns the original number.
The additive identity formula is expressed as $a + 0 = a,\; 0 + a = a$
Thus, $a = 6$
2. If $x \times 1 = 950$, then what is the value of x?
Solution:
Given: $x \times 1 = 950$
By multiplicative identity property, if 1 is multiplied by a number, it returns the original number.
The multiplicative identity formula is expressed as $a \times 1 = a$.
Thus, $x = 950$
3. Observe each equation and state whether the multiplicative identity property or the additive identity property is used.
a) $2 \times 8 = 16$
b) $1 + 0 = 1$
c) $0 + (\;-\; \frac{1}{2} ) = (\;-\; \frac{1}{2} )$
d) $-1 1 = \;-\;1$
Solution:
The multiplicative identity states that if a number is multiplied by 1 the resultant will be the number itself. It can be expressed as $a \times 1 = a$.
Additive identity, when added to a number, gives the same number as the result.
The additive identity formula is expressed as $a + 0 = a,\; 0 + a = a$.
From the given equations, the only equation that satisfies the multiplicative identity rule is
$\;-\;1 \times 1 = \;-\;1$.
$2 \times 8 = 16$ is not based on the multiplicative identity property.
From the given equations, the equations that satisfy the additive identity rules are
$1 + 0 = 1$ and $0 + (\;-\; \frac{1}{2}) = (\;-\; \frac{1}{2})$.
4. Which of the 2 equations illustrates the identity property of multiplication?
a) $20 \times 1 = 20$
b) $\;-\; 20 \times \;-\;1 = 20$
Solution:
a) $20 \times 1 = 20$
Yes, this equation illustrates the identity property of multiplication. The number 20, when multiplied by 1, gives the same result as the number itself, 20.
b) $(\;-\;20) \times (\;-\;1) = 20$
No, this equation does not illustrate the identity property of multiplication. The number $\;-\;20$, when multiplied by $\;-\;1$, gives 20, a different number.
5. If $35 + n = 35$, what is the value of n? State the property that you used.
Solution:
$35 + n = 35$ (given)
$n = 35 \;-\; 35$
$n = 0$
So, $35 + 0 = 35$
The property used here is the additive identity property.
Practice Problems on Additive Identity vs Multiplicative Identity
Additive Identity vs Multiplicative Identity - Definition With Examples
Which of the following is an example of multiplicative identity property?
Multiplicative Identity states that any number multiplied by 1 results in the number itself and is expressed as $a\times1 = a.$
Which of the following is the example of additive identity property?
An additive identity is a number that when added to any other number gives the sum as the number itself. The additive identity formula is expressed as $a + 0 = a,\; 0 + a = a.$
Use the multiplicative identity property to find the correct value of “a” if $a\times1 = \;-\;6$.
$a \times 1 = \;-\; 6$ (given). By multiplicative identity, if 1 is multiplied by a number, the product is the original number. So, since the product is $\;-\;6$, a is also $\;-\;6$.
$\;-\;6 \times 1 = \;-\;6$
What is the multiplicative identity element for real numbers?
The multiplicative identity of a real number is 1. When we multiply 1 by any real number, we get the same number.
For example, $30 \times 1 = 30$.
Which of the following numbers will make the equation, ___ $\times 1 = \frac{80}{100}$, true ?
By multiplicative identity property, if 1 is multiplied to a number, the product is the original number. Since the product is $\frac{80}{100}$, the missing number is also $\frac{80}{100}$.
$\frac{80}{100}\times1 = \frac{80}{100}$
Frequently Asked Questions on Additive Identity vs Multiplicative Identity
What is the difference between additive identity and the additive inverse of a number?
Additive inverse of a number, when added to the number, gives zero. The additive inverse of a number a is $–\; a$. It is expressed as $a + (\;-\; a) = 0$.
Additive identity, when added to a number, gives the same number as the result. The additive identity of a number is 0. The additive identity formula is expressed as $a + 0 = a$, $0 + a = a$
Is $-\;1$ a multiplicative identity?
No, -1 is not a multiplicative identity. A multiplicative identity is a number that when multiplied to any number gives the product as the number itself. If we multiply any number with -1, it will change the sign of the number. For example, $2 \;-\; 1 = \;-\;2$
Is additive identity applicable to negative integers?
Yes, the additive identity is applicable to negative integers as well. When we add 0 to any negative integer, the result of the addition will be the same original number. For example $0 + (\;-\; 5 ) = \;-\; 5$
Why is 0 not an identity of subtraction?
The number 0 is not an identity of subtraction as $a \;-\; 0 = a$, but $0 \;-\; a \neq a$.
What is the difference between multiplicative identity and multiplicative inverse?
When an element is multiplied by multiplicative identity, it gives the same number. Multiplicative identity is 1 and the formula is expressed as $a \times 1 = a$.Reciprocal of a number is called the multiplicative inverse. Multiplicative inverse of a is $\frac{1}{a}$. Multiplicative inverse of 4 is $\frac{1}{4}$. Multiplicative inverse of $\frac{2}{3}$ is $\frac{3}{2}$.























