Distributive Property – Definition, Examples, Facts, FAQs

What Is Distributive Property?

According to the distributive property, multiplying the sum of two or more addends by a number will give the same result as multiplying each addend individually by the number and then adding the products together.

To “distribute” means to divide something or give a share or part of something.

So what does distributive property mean in math? The distributive property describes how we can distribute multiplication over addition and subtraction.

Distributive Property Formula (Bold)

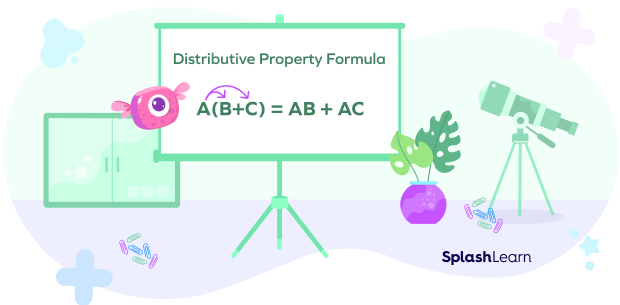
According to the distributive property, an expression of the form $A (B + C)$ can be solved as

$A (B + C) = AB + AC$.

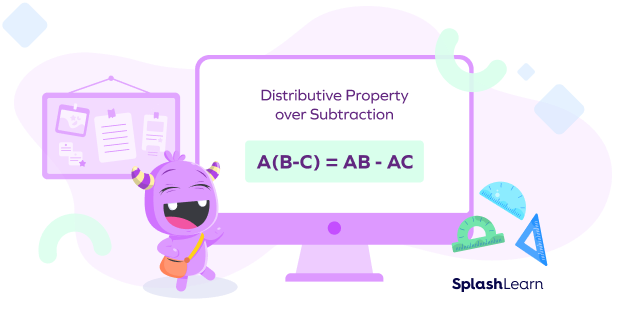
This property applies to subtraction as well. 

$A (B \;–\; C) = AB \;–\; AC$

Here, the multiplier A is ‘distributed’ in order to multiply it with B and C separately. 

(A, B, and C are any real numbers.)

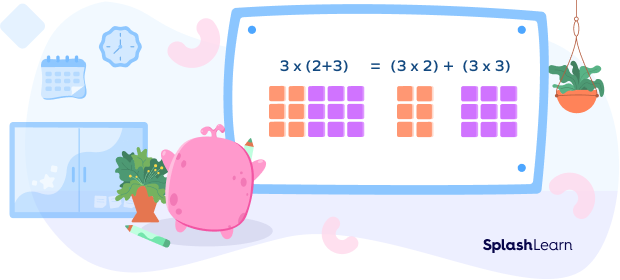
Let’s visualize the distributive property using multiplication arrays!

$3 \times (2 + 3)$ is represented by 3 rows and 5 columns. 

$3 \times 2$ is represented by 3 rows and 2 columns.

$3 \times 3$ is represented by 3 rows and 3 columns.

You can see that we broke down a big array into two smaller arrays using the distributive property.

Here’s an example of how the result does not change when solved normally and when solved using the distributive property:

Using order of operations	Using distributive property
$(1 + 2 + 3) \times 5$ $=6 \times 5$ $= 30$	$(1 + 2 + 3) \times 5$ $=(1 \times 5) + (2 \times 5) + (3 \times 5)$ $=5 + 10 + 15$ $= 30$

This property helps in making difficult problems simpler. You can use this property of multiplication to rewrite an expression by distributing or breaking down a factor as a sum or difference of two numbers.

Distributive Property: Definition

The distributive property is a fundamental property that defines how multiplication operation is distributed over addition and subtraction.

The distributive property is also called the distributive law of multiplication over addition and subtraction. 

Distributive Property of Multiplication over Addition

When we have to multiply a number by the sum of two numbers, we use the distributive property of multiplication over addition. 

Distributive Property of Multiplication over Addition:  $A (B + C) = AB + AC$

Distributive Property of Multiplication over Subtraction

The distributive property of multiplication over subtraction is equivalent to the distributive property of multiplication over addition, except for the operations of addition and subtraction.

Distributive Property of Multiplication over Subtraction:  $A (B \;-\; C) = AB \;-\; AC$

$A(B \;−\; C)$ and $AB \;−\; AC$ are equivalent expressions.

Consider these distributive property examples below.

Verification of the Distributive Property

We can verify the distributive property by solving both LHS and RHS. 

Example: Solve the expression $2 (4 \;–\; 3)$. 

Using the distributive law of multiplication over subtraction, we have

$2 \times (4 \;–\; 3) = (2 \times 4) \;–\; 2 \times 3 = 8 \;–\; 6 = 2$

Again, if we try to solve the expression with the order of operations or PEMDAS, we’ll have to subtract the numbers in parentheses, then multiply the difference with the number outside the parentheses, which implies:

$2 \times (4 \;–\; 3)=2 \times 1 = 2$

The distributive property of subtraction is verified since both techniques give the same result.

How to Use the Distributive Property

There are three simple steps to use the distributive property.

Step 1: Distribute the multiplier (the number outside the parentheses).

Step 2: Find the individual products.

Step 3: Add or subtract.

Let’s understand how to use the distributive property with the help of examples.

Examples of distributive property of multiplication over addition and subtraction:

Example 1: Solve the expression: $6 \times (20 + 5)$ using the distributive property of multiplication over addition. 

Let’s use the property to calculate the expression $6 \times (20 + 5)$, the number 6 is spread across the two addends. To put it simply, we multiply each addend by 6 and then the products can be added.

$6 \times 20 + 6 \times 5 = 120 + 30 = 150$

Example 2: Solve the expression $2 \times (2 + 4)$ using the distributive law of multiplication over addition.

$2 \times (2 + 4) = 2 \times 2 + 2 \times 4 = 4 + 8 = 12$

If we try to solve this expression using the PEMDAS rule, we’ll have to add the numbers in parentheses and then multiply the total by the number outside the parentheses. This implies:

$2 (2 + 4) = 2 \times 6 = 12$

Thus, we get the same result irrespective of the method used.

Example 3: Solve the expression $6 \times (20 \;–\; 5)$ using the distributive property of multiplication over subtraction.

Using the distributive property of multiplication over subtraction, we get

$6 \times (20 \;–\; 5) = 6 \times 20 \;–\; 6 \times 5 = 120 \;–\; 30 = 90$

Distributive Property of Division

We can use the distributive law of division by distributing the dividend (or breaking down the dividend) into the sum of two numbers (partial dividends), such that both the numbers are completely divisible by the divisor. 

Example: $132 \div 6$

132 can be broken down as $60 + 60 + 12$, thus making division easier. Note that 60 and 12 both are divisible by 6.

$132 \div 6 = (60 + 60 + 12) \div 6$

$132 \div 6 = (60 \div 6) + (60 \div 6) + (12 \div 6)$

$132 \div 6 = 10 + 10 + 2$

$132 \div 6 = 22$

We cannot break $132 \div 6$ as $(50 + 50 + 32)\div 6$ since 50 and 32 are not divisible by 6.

Also, we cannot break the divisor:  $132 \div (4 + 2)$ will give you the wrong result. 

Facts about Distributive Property

Conclusion

In this article, we learned about the distributive property, formulas, when to use the distributive property, and also how to use distributive property in complex equations and problems. Let’s solve a few examples and practice problems based on the distributive property.

Solved Examples on Distributive Property

Example 1:  Solve $(5 + 7 + 3) 4$.

Solution: Using the distributive property of multiplication over addition,

$A \times (B + C) = AB + AC$

$(5 + 7 + 3) \times 4$

$= ( 5 \times 4) + (7 \times 4) + (3 \times 4)$

$= 20 + 28 + 12$

$= 60$

We can verify it as:

$(5 + 7 + 3) \times 4 = 15 \times 4 = 60$

Example 2: Solve the following using the distributive property: $\;−\;2 (\;−\;x \;−\; 7)$. 

Solution: Using the distributive property,

$A (B \;–\; C) = AB \;–\; AC$

$\;−\;2 (\;−\;x \;−\; 7) = (\;−\;2)(\;−\;x) \;−\; (\;−\;2)(7)$

     $= 2x \;−\; (\;−\;14)$

     $= 2x + 14$

Example 3: Which property does the equation $3 (4 \;−\; 9) = (3 \times 4) \;−\; (3 \times 9)$ show?

Solution:

Given equation: $3 (4 \;−\; 9) = (3 \times 4) \;−\; (3 \times 9)$

Comparing it with the distributive property formula for multiplication over subtraction $A (B \;-\; C) = AB \;-\; AC$, we get that the above equation shows the distributive property of multiplication over subtraction.

Practice Problems on Distributive Property

Frequently Asked Questions on Distributive Property