Introduction of Multiplying Decimals
Multiplication is an essential life skill that everyone needs to know. Multiplying decimals is a more advanced multiplication skill, which is an upskill compared to simple whole-number multiplications. Acquainting children with something that’s a little complex—like decimal multiplications—will train them to undertake more complicated math concepts.
Multiplying decimals can seem complicated and daunting to young minds. As a parent or teacher, how can you bridge this gap between dislike and a vital math skill? Growing interest and curiosity can counter dislike or fear of a subject. SplashLearn is a platform built to assist young minds in comprehending complex mathematical concepts that align with the school curriculum through games.
Yes, you read it right. After all, what is more tempting and interactive to kids than games? SplashLearn games are a fun and innovative way to teach theories and concepts to children, which would otherwise be a tedious enterprise for them.
Recommended Games
Multiplying Decimals with Whole Number
Multiplying a decimal number with a whole number is similar to multiplying whole numbers. However, the decimal places in one of the numbers can make it seem difficult. So, let us see how we can simplify the process for you.
Step 1: Forget about the decimals. Yes, they don’t play any role while you are performing the actual operation. Now, multiply the two numbers as you would do with whole numbers.
Step 2: Count the number of digits after the decimal point.
Step 3: Place the decimal point in the product leaving the same number of digits from the right as the decimal places in the factor.
Pro Tip: Always remember that the product after multiplication must have the same number of decimal places as the factors.
Now, let us look at examples of multiplying decimals:
Example: Find the product of $0.2 \times 3$

Step 1: We take the two numbers without considering the decimal, i.e., 2 and 3. We multiply them. So, we get $2 \times 3 = 6$.
Step 2: We count the number of digits after the decimal point. Here we have just one digit after the decimal.
Step 3: We place the decimal point in the product leaving behind one digit from the right. Since the product is 6, we have to place the decimal before it.
So, the answer will be 0.6.
Example: Find the product of $0.4 \times 3$
Similarly to find the product of 0.4 and 3, multiply the numbers without decimals i.e $4 \times 3 = 12$.
Now there’s one digit after decimal point so let’s place the decimal point in the product leaving behind one digit from the right : 1.2
So $0.4 \times 3 = 1.2$
Recommended Worksheets
Multiplication of Two Decimal Numbers
While multiplying two decimal numbers, you must consider the decimal places of both factors.
Step 1: Ignore the decimal places initially. Perform the multiplication of the given numbers.
Step 2: Counter the number of decimal places in both the factors and calculate the sum of the same.
Step 3: Place the decimal point from the right end according to the number of decimal places you calculated in step 2.
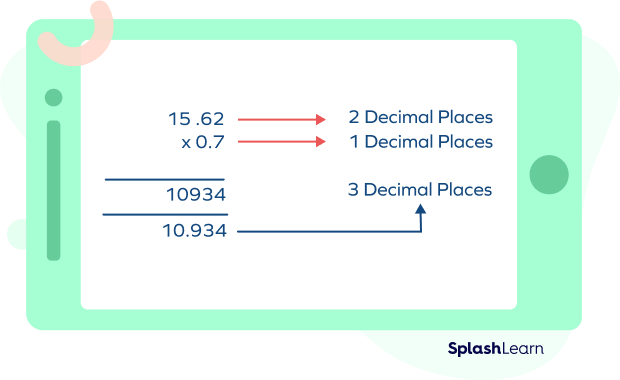
Example: Find the product of $15.62 \times 0.7$
Step 1: We ignore the decimals and multiply the given numbers.
$1562 \times 7 = 10934$
Step 2: We count the number of decimal places in both the numbers and calculate their sum.
15.62 has 2 decimal places, and 0.72 has 1 decimal place. Therefore, the sum of the decimal factors is 3.
Step 3: We place the decimal in the product leaving behind three digits from the right.
So, $15.62 \times 0.7 = 10.934$
Multiplication of Decimals by 10, 100, and 1000
When we multiply a decimal number by 10, the decimal shifts to the right of the number by one place.
For example, $15.2705 \times 10 = 152.705$

Here, only one change takes place in the solution: the decimal shift from its position in 15.2705 to one place to the right. So, we get 152.705 as the answer.
When we multiply a decimal number by 100, the decimal shifts to the right of the number by two places.
For example, $15.2705 \times 100 = 1527.05$
When we multiply a decimal number by 1000, the decimal shifts to the right of the number by three places.
For example, $15.2705 \times 1000 = 15270.5$
So, when we have to multiply a decimal number by 10, 100, or 1000, we shift the decimal to the right of the number according to the number of zeros behind 1.
Let us consider a few examples:
- $53.5 \times 10 = 535$
- $469.127 \times 10 = 4691.27$
- $398.16 \times 100 = 39816$
- $826.3 \times 100 = 82630.0$
- $0.137 \times 100 = 13.7$
Solved Examples
1) Find the product of $9.2 \times 0.08$.
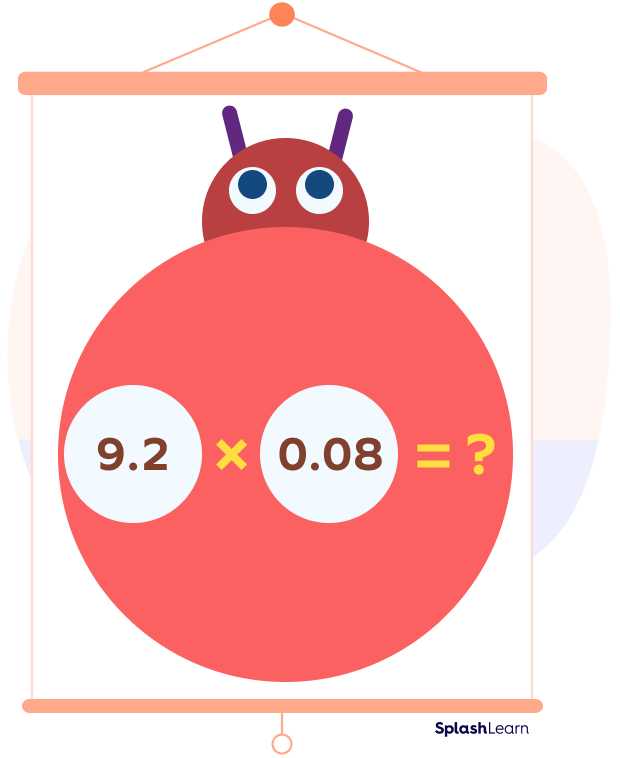
Solution:
Step 1: We ignore the decimals and multiply the given numbers.
$92 \times 8 = 736$
Step 2: We observe that 9.2 has 1 decimal place, and 0.08 has 2 decimal places. Therefore, the sum of the decimal factors is 3.
Step 3: We place the decimal in the product leaving behind three digits from the right.
So, $9.2 \times 0.08 = 0.736$
2) Find the product of $20.1 \times 6.0$.

Solution:
Step 1: We ignore the decimals and multiply the given numbers.
$201 \times 60 = 12060$
Step 2: We observe that 20.1 has 1 decimal place, and 6.0 has 1 decimal place. Therefore, the sum of the decimal factors is 2.
Step 3: We place the decimal in the product leaving two digits from the right.
So, $20.1 \times 6.0 = 120.60$
3) Find the product of $36 \times 0.5$.
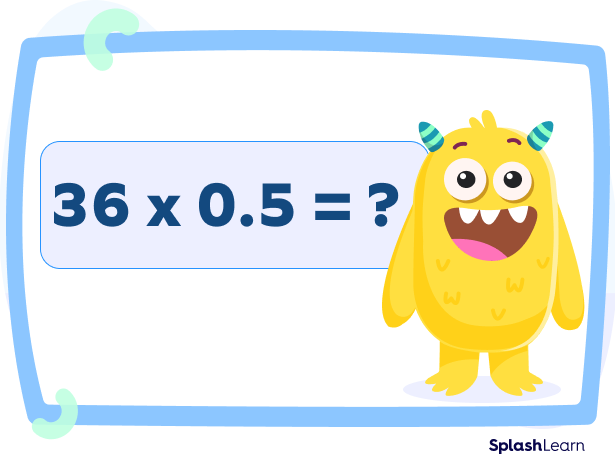
Solution:
Step 1: We ignore the decimal and multiply the given numbers.
$36 \times 5 = 180$
Step 2: We observe that 0.5 has 1 decimal place.
Step 3: We place the decimal in the product leaving behind one digit from the right.
So, $36 \times 0.5= 18.0$
Practice Problems
Multiplying Decimals - Definition with Examples
What will you get if you multiply 341.58 with 4?

We ignore the decimal and multiply the given numbers.
We get $34158 \times 4 = 136632$
Since $341.58$ has two decimal places, we place the decimal in the product leaving two digits from the right.
So, the answer is $1366.32$.
Which one of the following is the right answer for the given multiplication problem? $64 \times 8.3$
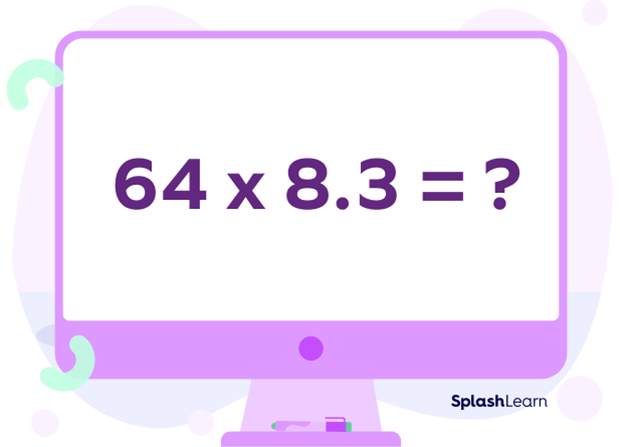
We ignore the decimal and multiply the given numbers.
We get $64 \times 83 = 5312$
Since $8.3$ has one decimal place, we place the decimal in the product leaving behind one digit from the right.
So, the answer is $531.2$
Find the product of $5.27 \times 3.9$.

We ignore the decimals and multiply the given numbers.
We get $527 \times 39 = 20553$
Since 5.27 has two decimal places and 3.9 has one decimal place, we place the decimal in the product leaving behind three digits from the right.
So, the answer is $20.553$.
Frequently Asked Questions
How can we multiply decimals by 10 and 100?
While multiplying a decimal by 10, shift the decimal to the right by one place. And when multiplying a decimal by 100, shift the decimal point by two places.
For example, $4.89 \times 10= 48.9$ and $3.4 \times 100 = 340$
What is the rule for multiplication of decimals?
There is no particular rule for decimal multiplication. First, multiply the given numbers as you would do with whole numbers. Then, calculate the sum of decimal places in both the factors and apply the same to the answer.
Will multiplying decimals always give a greater product?
No. If one of the decimal numbers is less than 1, then the product will be smaller. But if both decimal numbers are greater than 1, then the product will also be greater than the numbers.




































