In elementary mathematics, the four basic arithmetic operations are addition, subtraction, multiplication, and division. Division is the method where we try to distribute a certain number of things into equal parts. For example, Dividing 20 apples among 5 people means that we have to distribute the 20 apples in such a way that all the 5 people have the same number of apples.
What is Dividend?
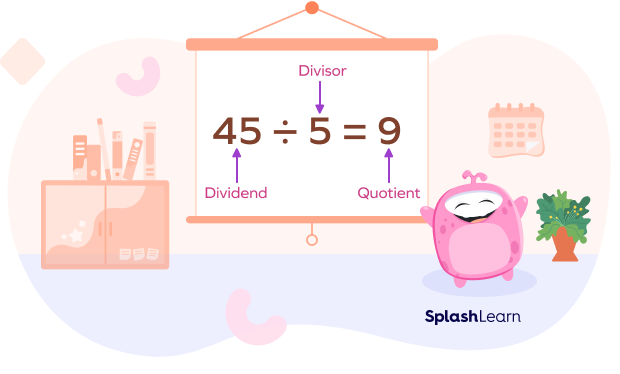
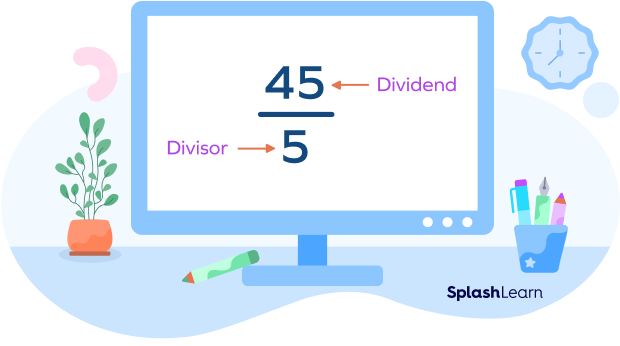
In a division problem, the number that is to be divided or distributed into a certain number of equal parts is called the dividend. As in the example above, when we are dividing 20 apples into 5 people, the dividend is the number 20; and the number 5 is called the divisor.
When the dividend is totally divisible by the divisor, the number so gained is called the quotient. While dividing 20 apples between 5 people, we see that each person gets 4 apples. We write this in equation form as
20 ÷ 5 = 4.
Here, the number 4 is the quotient.
In case when the dividend is not totally divisible by the divisor, we get some remaining amount.
For example, if we were to divide 21 apples among 5 people, we would only be able to give 4 apples to each person and then we would be leftover with 2 apples.
This number 2 which is left after the division is called the remainder.
Recommended Games
How to identify Dividend
There are several ways to express a division problem.
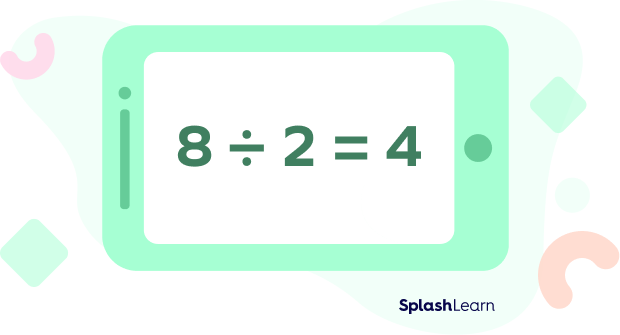
- The first one is the division equation. In a division equation(as given below), the number appearing right before the division symbol is called the dividend.
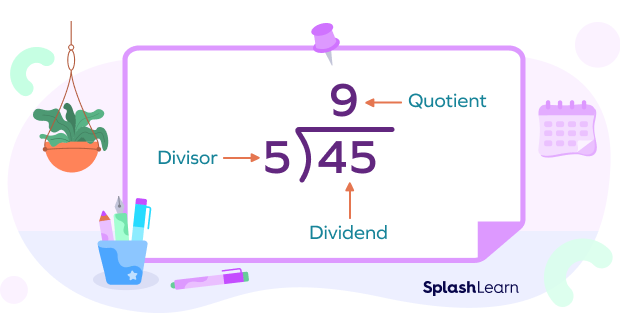
- In a long division method, the number that appears inside the long division symbol is called the dividend.
- In fraction notation, the number that appears on the top is the dividend.
Recommended Worksheets
Relation between the Dividend, Divisor, Quotient, and Remainder
This relation between the four terms of division operation is given by the formula
Dividend = Divisor × Quotient + Remainder
This relation can be used to verify a division process or can also be sued to find the dividend when only the divisor, quotient, and remainder are given.
For example, a certain division problem has the quotient as 4, remainder as 2, and divisor as 5.
The dividend for the problem can be found by using the formula
Dividend = Divisor × Quotient + Remainder
Dividend = 5 × 4 + 2 = 20 + 2 = 22.
Some Facts about the Dividend
- The word dividend is a derivative of the Latin word dividendum, which means a thing to be divided.
- If the dividend and the divisor are the same, the quotient is always 1.
- If the dividend is the, then the quotient would always be 0.
- A division equation with 0 dividend and 0 divisor is not valid.
Divide 0 apples among 0 people. Wait.. what?
- If the dividend is smaller than the divisor, the quotient would always be a decimal number.
Solved Examples
- If the quotient is 6, the divisor is 9, and the remainder is 2, then find the dividend.
Solution: Given: Divisor = 9, Quotient = 6, Remainder = 2
Dividend = Divisor × Quotient + Remainder
Dividend = 9 × 6 + 2 = 54 + 2 = 56.
- Solve the problem and determine the dividend: A school planned to take their students for a picnic. 90 children registered their names, and 3 buses were booked. Calculate the number of children accommodated in each bus? Form the division fact for the above problem, and determine the dividend.
Solution: To calculate the number of children in each bus, you need to divide the number of children among the number of buses available.
So, you will have to divide 90 by 3 –
903=30
Thus, each bus was able to accommodate 30 children.
Therefore, the dividend is 90, the divisor 3, and the quotient 30.
- Find the dividend when the divisor is 4, quotient is 0.5.
Solution: Given: Divisor = 4, Quotient = 0.5, Remainder = 0
Dividend = Divisor × Quotient + Remainder
Dividend = 4 × 0.5 + 1 = 2 + 0 = 2.
Practice Problems
Dividen
Find the dividend in the division fact: 90 ÷ 9 = 10.
The number that is being divided is the dividend i.e. 90.
What is the dividend: 1537?
The number that is being divided is the dividend i.e. 153
Find the dividend if the divisor is 5, quotient is 3 and remainder is 1.
On applying the formula Dividend = Divisor Quotient + Remainder, we get Dividend = 16.
20 chocolates are distributed among 4 kids in such a way that each kid gets 5 chocolates. What is the dividend in this example?
The number that is being divided or distributed is the dividend i.e. 20.
Frequently Asked Questions
Can 0 be a dividend?
Yes, however, dividing 0 by any number always results in 0.
How to check if the division is correct?
This relation can be used to verify a division process or can also be used to find the dividend when only the divisor, quotient, and remainder are given.
Dividend = Divisor × Quotient + Remainder
How do you find the dividend when the remainder is zero?
When the remainder is zero, you can find the dividend by using this formula:
Dividend = Divisor x Quotient
Can the divisor be greater than the dividend?
Yes, the divisor can be greater than the dividend. In that case, the quotient will be a decimal number. For example, 85 ÷ 1000 = 0.085.