Multiplicative Inverse – Definition, Examples, Practice Problems

What Is Multiplicative Inverse?

The meaning of the word “inverse” is something opposite in effect. The multiplicative inverse of a number is a number that, when multiplied by the given number, gives 1 as the product. By multiplicative inverse definition, it is the reciprocal of a number. 

The multiplicative inverse of a number “a” is represented as a^-1 or $\frac{1}{a}$.  

Multiplicative Inverse Property

The multiplicative inverse property states that if we multiply a number with its reciprocal, the product is always equal to 1. The image given below shows that $\frac{1}{a}$ is the reciprocal of the number “a”.

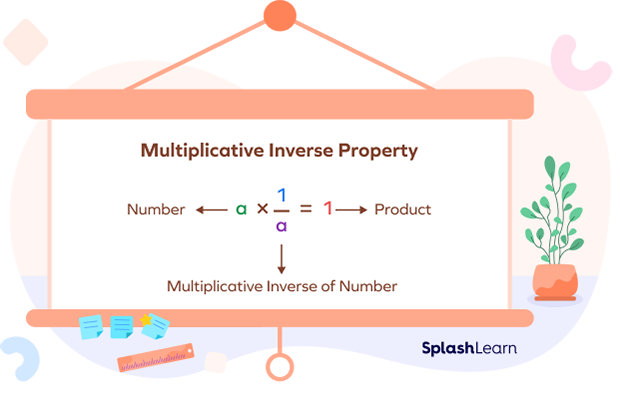
A pair of numbers, when multiplied to give product 1, are said to be multiplicative inverses of each other. Here, a and $\frac{1}{a}$ are reciprocals of each other. 

How to Find the Multiplicative Inverse?

Consider that we have seven apples. To make them into groups of 1 each, we need to divide them by 7. Since division is the reverse process of multiplication, dividing by a number is equivalent to multiplying by the reciprocal of the number.

Thus, 7 ÷ 7 = 7 × $\frac{1}{7}$ = 1

Here, $\frac{1}{7}$ is called the multiplicative inverse of 7. 

Let us understand the multiplicative inverse of different types of numbers like natural numbers, integers and fractions.

Natural Numbers

The numbers that are used for counting, such as 1, 2, 3, and so on, are known as natural numbers. The reciprocal of “a” is $\frac{1}{a}$.

For example, if we multiply 7 by $\frac{1}{7}$, we get $7\frac{1}{7}=1$. So, the multiplicative inverse of 7 is $\frac{1}{7}$. 

Integers

The reciprocal of a positive integer “a” is $\frac{1}{a}$. Negative integers lie on the left side of zero on a number line and their value is always less than 0. Negative integers have minus (-) sign in front of them. The product of any integer and its reciprocal must be 1. The reciprocal of a negative number will be a negative number. For example: If the number is -2, then its reciprocal will be $-\frac{1}{2}$ not $\frac{1}{2}$, as  $-2 \times \left[-\frac{1}{2}\right] = 1$

Fractions

To find the reciprocal of a fraction we can just flip it over. The reciprocal of any fraction $\frac{a}{b}$ is $\frac{b}{a}$, because $\frac{a}{b}\times\frac{b}{a}=1$.

Unit Fractions

Unit fraction is the fraction in which the numerator is 1 irrespective of the number in the denominator. The reciprocal of unit fraction $\frac{1}{x}$ is x, a whole number. For example, reciprocal or multiplicative inverse of ¼ is 4. 

Mixed Fraction

A mixed fraction is a combination of a whole number and a proper fraction. For example: $2\frac{3}{7},5\frac{4}{5}$, etc.

In order to find the reciprocal of a mixed fraction, we convert it to an improper fraction and then find its reciprocal. 

For example, to find the reciprocal of $2\frac{3}{7}$,  we convert $2\frac{3}{7}$ to improper fraction, that is $\frac{17}{7}$. 

Since the reciprocal of $2\frac{3}{7}$ or $\frac{17}{7}$ is $\frac{7}{17}$.

Fun Facts

Conclusion

In this article, we learned about the reciprocal of different types of numbers. To read more such informative articles on other concepts, do visit our website. We, at SplashLearn, are on a mission to make learning fun and interactive for all students.

Solved Examples on Multiplicative Inverse

Example 1: What is the multiplicative inverse of -100?

Solution: The multiplicative inverse of -100 is -$\frac{1}{100}$. 

Example 2: The reciprocal of a number is $2\frac{3}{5}$. Find the number.

Solution: A pair of numbers when multiplied to give product as 1, they are said to be reciprocals of each other.

So, the reciprocal of $2\frac{3}{5}$ or $\frac{13}{5}$ is the original number. Since the reciprocal of $\frac{13}{5}$ is $\frac{5}{13}$, the original number is $\frac{5}{13}$.

Example 3: What is the multiplicative inverse of $\frac{2}{3} + \frac{3}{2}$?

Solution: To find the reciprocal, we need to simplify the expression first.

$\frac{2}{3} + \frac{3}{2} = \frac{13}{6}$The reciprocal of $\frac{13}{6}$ is $\frac{6}{13}$.

Practice Problems on Multiplicative Inverse

Frequently Asked Questions on Multiplicative Inverse