Subtracting Mixed Numbers
Fractions which are more than a whole are known as mixed fractions or mixed numbers.
For instance: Nicholas has 3 chocolates, and each chocolate has 3 bars. He eats 7 bars of chocolate, which means 2 whole chocolates and 1 bar of the third chocolate.
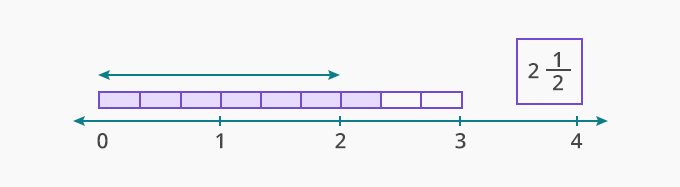
Real-life examples of Subtracting mixed numbers:

Recommended Games
How to Subtracting mixed numbers?
Case 1: Subtracting mixed numbers with same denominators
Follow the given steps to subtract mixed numbers with same denominators:
Step 1– Subtract the wholes.
Step 2– Convert the fractions into improper fractions.
Step 3– Subtract the fraction.
Step 4– Change the improper fraction into a mixed number if needed.
Step 5– Write the mixed number with wholes and the fraction.
Example 1: 5 7⁄3 – 3 2⁄3
Step 1 – Subtract 3 from 5.
5 – 3 =2
Step 2 – Subtract the fractions:
7⁄3 – 2⁄3
Step 3 – Write the fraction with whole.
5 7⁄3 – 3 2⁄3 = 2 5⁄3
Case 2: Subtracting mixed numbers with different denominators
Follow the given steps to subtract mixed numbers with different denominators:
Step 1– Convert the mixed numbers into improper fractions.
Step 2– Find the common multiple of both the denominators.
Step 3– Convert the fractions as common denominators.
Step 4– Solve the fractions.
Step 5– Convert the fraction as a mixed number.
Example 2: 6 1⁄2 – 1 3⁄4
Step 1– Convert the mixed numbers into improper fractions.
1 3⁄2 – 7⁄4
Step 2– Find the common multiple of both the denominators 2 and 4.
The common multiple of 2 and 4 is 4.
Step 3– Convert the fractions as common denominators.
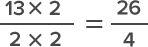
Step 4– Solve the fractions
26⁄4 – 7⁄4 = 19⁄4
Step 5– Convert the fraction as a mixed number.
19⁄4 = 4 3⁄4




































