Decagonal Prism: Introduction
Before we discuss decagonal prism, let’s recall some important concepts and definitions. A prism is a three-dimensional figure made up of two kinds of faces: bases and lateral faces. The bases of a prism are polygons that form the top and bottom of the prism. The two bases of a prism are identical polygons like a triangle, a square, a rectangle, or a hexagon. A prism is named for the shape of its base.
These can be triangles, squares, or any other polygon. The lateral faces connect the base and the top and are usually parallelograms.
For instance, a pentagonal prism is called so because its two bases are regular pentagons.
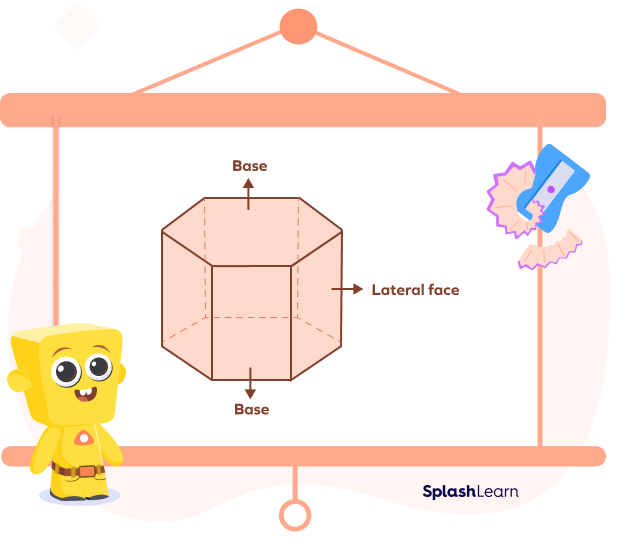
Similarly, a hexagonal prism has 2 regular hexagons as its bases and 6 faces.
Based on this, can you now figure out what a decagonal prism is? Let’s find out!
What Is a Decagonal Prism?
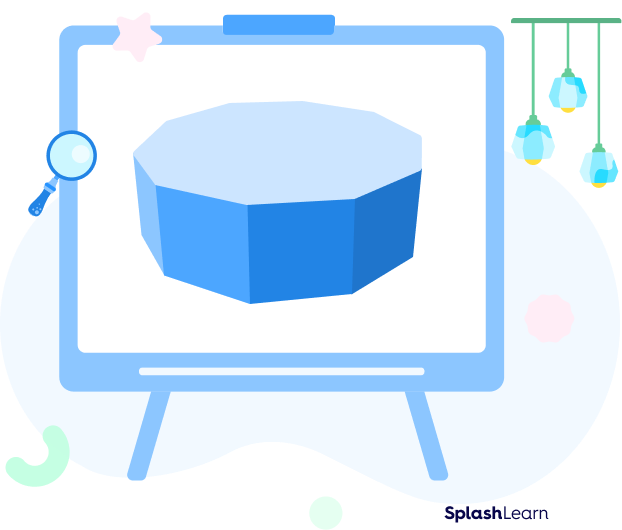
The decagonal prism is formed by two regular decagons as its bases and 10 squares as its other faces. Thus, the top of the decagonal prism is a regular decagon, so is the bottom face.
In other words, it is a three-dimensional figure with two identical decagonal faces on the top and bottom, which are regular decagons, and ten faces, which are squares.
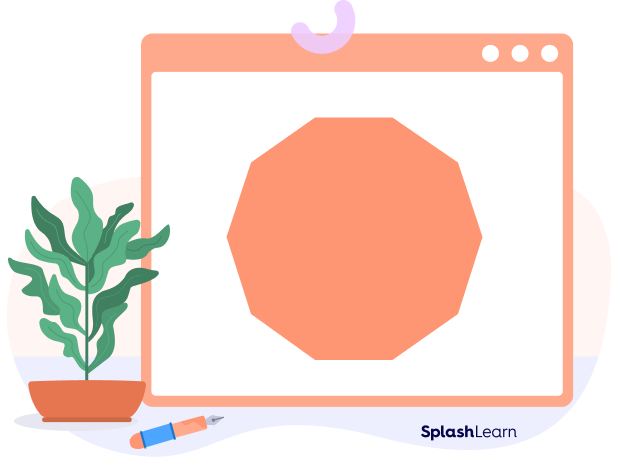
What Is a Regular Decagon?
A decagon is a polygon made up of 10 sides. A regular decagon is a decagon in which all the 10 sides are equal.
In a decagonal prism, the 10 square faces and the 2 decagon bases make up 12 faces in total.
What Kind of 3D Figure Is a Decagonal Prism?
A decagonal prism is a polyhedron.
A polyhedron is a three-dimensional figure made up of polygons. It has flat faces, straight edges, and vertices.
Some examples of polyhedrons are a cube, prism, and pyramid.

In the picture above, the pyramid and cube are polyhedrons.
A decagonal prism is made up of 10 squares and 2 hexagons, so it is a polyhedron.
How to Calculate the Volume of a Decagonal Prism?
The volume of a prism tells you how much space it occupies.
The volume of any prism can be calculated with the following formula:
Volume $=$ surface area of base $\times$ height of the prism
The surface area of base refers to the surface area of the decagonal base. The height is the column of the prism that connects the two bases.
The value you get when you multiply the base with the height will be in cubic units like cubic inches, cubic yards, cubic feet, or cubic meters. These units measure three-dimensional space or volume.
Solved Examples
1. For a decagonal prism, the surface area of base $= 15$ sq. ft, height $= 20$ ft. What is the volume?
Solution:
Volume of decagonal prism $=$ Surface area of base $\times$ Height
Volume of decagonal prism $= 15 \times 20 = 300$ square feet
2. In a decagonal prism, the base has the surface area of 1 square inches, height $= 1$ inch. What is the volume ?
Solution:
Volume $=$ Surface area of base $\times$ Height
Volume $= 1$ square inches $\times 1$ inch
Volume $= 1$ cubic inches
3. The base of a decagonal prism has a surface area of 277 inch². The height is 10 inches. Calculate the volume.
Solution: Volume $=$ surface area of base $\times$ height
$= 277 \times 10$
$= 2770$ $\text{inch}^{3}$
4. The volume of a decagonal prism is $1108$ $\text{inch}^{3}$. The surface area of the base is 123 inch². What is the height?
Solution: Volume = surface area of base $\times$ height
$= 1108 = 123 \times$ height
Height $= 1108 / 123 = 9$
So, the height is 9 inches.
Practice Problems
Decagonal Prism - Definition with Examples
What are the two bases of a decagonal prism?
The two bases of a decagonal prism are decagons with equal sides, which are called regular decagons.
The base of a decagonal prism has a surface area of 377 inch². The height is 9 inches. Calculate the volume.
Volume $=$ surface area of base $\times$ height
$= 377 \times 9$
$ = 3393$ $\text{inch}^{3}$
The volume of a decagonal prism is $377,100 \text{cm}^{3}$. The surface area of the base is $7394 \text{cm}^{2}$. What is the height?
Volume $=$ surface area of base $\times$ height
$377100 = 7394 \times$ height
Height $= 377100 \div 7394 = 51$
So the height is $51$ $\text{cm}$.
Frequently Asked Question
How many edges and vertices does a decagonal prism have?
A decagonal prism has 30 edges and 20 vertices. 10 vertices of the decagon at the top and 10 vertices of the decagon at the bottom make the 20 vertices of the decagonal prism.
What are the other kinds of prisms?
Other kinds of prisms include triangular prisms, rectangular prisms, pentagonal prisms, and hexagonal prisms. These prisms are named after the polygons that form their bases, such as triangles, rectangles, pentagons, and hexagons.
Why is a decagonal prism said to be a dodecahedron?
A dodecahedron is a polyhedron that has 12 faces. A decagonal prism has 12 faces in total (10 sides and 2 bases), so it is a dodecahedron.
The most common example of a dodecahedron is the regular dodecahedron, which has 12 faces that are all regular pentagons.
















