Monomial – Definition, Degree, Parts, Examples, Facts, FAQs

What Is a Monomial in Math?

A monomial is a polynomial that contains only a single non-zero term. 

“Mono” means one. When polynomials are classified on the basis of the number of terms, the polynomials with only a single term are called monomials. This single term can be a number (a constant), a variable, or a product of multiple variables with a coefficient. 

The coefficient can be any real number. There won’t be any operator present in a monomial.

Note that the degree of a monomial need not be 1. The exponents of variables cannot be negative or fractional. The exponent of all variables must be a whole number (non-negative integer).

Monomial examples:

• $5a^{2}$

• $\;-\;3pq$

• $\frac{-1}{2}xy^{2}zpq$

• $4x^{2}y$

• $xyz$

• $-7\;x^{4}y^{8}z^{3}$

Monomial: Definition

A monomial can be defined as a polynomial with a single non-zero term.

Parts of a Monomial

Different parts of a monomial are as follows:

• Variable: the letters present in the expression
• Coefficient: the number attached with the variable 
• Degree: the sum of exponents of all the variables present
• Literal part: the alphabets and their exponents 

Monomial	Variable	Degree	Literal part
$5x^{3}$	x	3	$x^{3}$
$-6x^{2}yz^{3}$	x,y,z	6	$x^{2}yz^{3}$
$9xy^{7}$	x,y	8	$xy^{7}$
8	No variable	0	–

How to Find Monomials

You can identify a monomial easily using the following points: 

1. The expression must have a single term, which implies that the combination of alphabets (variables) must not be separated by any operator.

2. The exponents must always be positive (a whole number). The exponents cannot be fractions.

For instance, $(2x + y), (3p \;-\; t), x^{\frac{1}{2}}$ are not monomials.

How to Find the Degree of a Monomial

The degree of a polynomial will always be a positive integer owing to the rule of monomials to contain positive exponents. The power of variables is summed up to calculate the degree of the polynomial as depicted in the diagram. Given below are the steps to finding the degree of a monomial:

Step 1: Identify the variables and their exponents. 

Step 2: Add all the exponents.

Step 3: The sum represents the degree. 

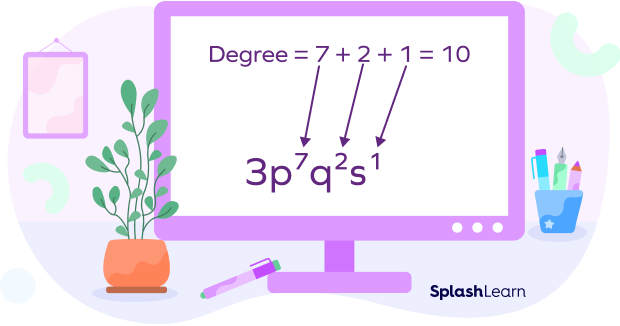
Example: $3p^{7}q^{2}s$ can be written as  $4p^{7}q^{2}s^{1}$.

Factoring Monomials

Factoring monomials simply means writing the coefficients and the variables in the factored form. In other words, monomial factorization is a way of expressing a given monomial as a product of two or more monomials.

How to Factor a Monomial

To factor monomials, we factor the coefficients and the literal part (variables) separately. Factorization refers to separating the terms into constituents whose product is the original term. 

Example 1:  $4p^{2}q^{3}s = 2 \times 2 \times p \times p \times q \times q \times q \times s$ 

Example 2: $15xy^{2}z^{3} = 3 \times 5 \times x \times y \times y \times z \times z \times z$

Monomials, Binomials, Trinomials

The polynomial can have only a finite number of terms. We can classify polynomials based on the number of terms. A single-term polynomial is called a monomial. Polynomials with two terms are called binomials. Polynomials with three terms are called trinomials. 

Monomial	Binomial	Trinomial
A polynomial with only one term.	A polynomial with two terms.	A polynomial with three terms.
$4p^{5}q^{3}s$	$4p^{5}q^{3} + s$	$4p^{5}\;-\;q^{3} + s$

Operations on Monomials

Addition of two monomials having the same literal part will result in a monomial.

Example:  $4p^{5}q^{3}s + 10p^{5}q^{3}s =   14p^{5}q^{3}s$

Subtraction of two monomials having the same literal part will result in a monomial.

Example:  $4p^{5}q^{3}s \;-\; 10p^{5}q^{3}s =  \;-\;6p^{5}q^{3}s$

Multiplication of two monomials is always a monomial.

Example:  $4p^{5}q^{3}s \times 10p^{5}q^{3} s =   40p^{10}q^{6}s^{2}$

To divide two monomials having the same variables, we use the laws of indices (rules of exponents).

Example: $\frac{40p^{10}q^{6}s^{2}}{4p^{5}q^{3} s} = 10p^{5}q^{3} s$

Facts about Monomials

Conclusion

Monomials are polynomials categorized based on terms. They contain one term with varying degrees calculated by summing up the exponents. They can comprise single or multiple variables. The factorization and other operations of monomials are performed similarly to general mathematics. 

Solved Examples on Monomials

1. State the parts of a monomial  $10a^{2}b^{2}$.

Solution: 

The parts of a monomial are coefficient, variable, degree and literal part. 

Coefficient: 10

Literal part: $a^{2}b^{2}$

Variable: a, b

Degree: $2 + 2 = 4$

2. Identify the monomials, binomials, and trinomials from the following polynomials.

1. $x^{2}y$
2. $a^{2} + b^{2}$ 
3. $p^{3}q^{2} \;-\; s^{2}t^{2}$ 
4. $a^{2} + b^{2} + 2ab$

Solution: 

Monomial: $x^{2}y$

Binomials: $a^{2} + b^{2} ,  p^{3}q^{2} \;-\; s^{2}t^{2}$

Trinomial:  $a^{2} + b^{2} + 2ab$

3. What is the degree of the given monomials? 

i) $5x^{3}$ 

ii) $x^{2}y$

Solution: 

i) Polynomial $= 5x^{3}$

Degree $= 3$

ii) Polynomial $= x^{2}y$

Degree $= 2 + 1 = 3$

Practice Problems on Monomials

Frequently Asked Questions about Monomials