Multiplication is one of the most basic elementary arithmetic operations that a student learns about while growing up. In elementary mathematics, multiplication is a more advanced way of adding a number multiple times. The very foundation of multiplication lies with the concept of repeated addition and hence the operation of multiplication follows the same properties as the addition operator. One of these properties is the associative property of multiplication.
What is the Associative Property of Multiplication?
To “associate” means to connect or join with something. The associative property of multiplication says that while multiplying three numbers, regardless of the way the numbers are grouped, the end result will always be the same.
Let’s try to understand the associative property of multiplication with an example:
Let’s try multiplying the numbers 2, 3, and 5.
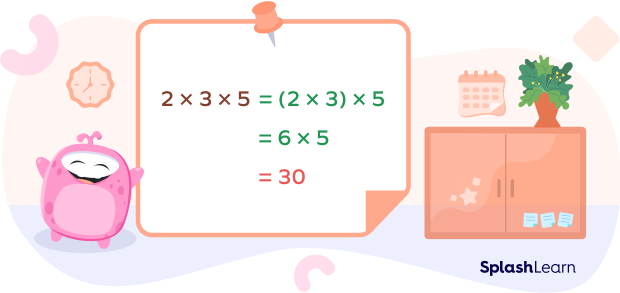
Now, we can multiply these numbers in different ways.
We could first multiply 2 and 3 and then multiply their product with 5.
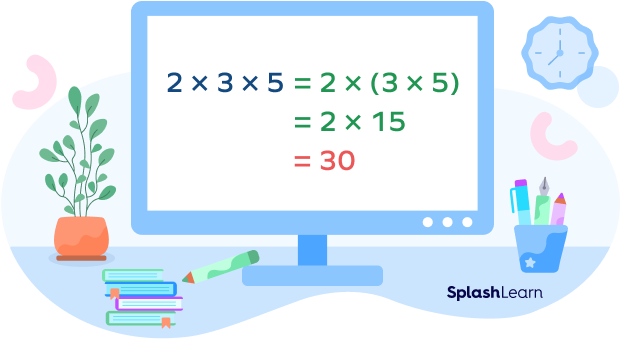
Or we could multiply 3 and 5 first and then multiply the product of these two numbers with
2.
As we can see, the product in both the cases is the same. This property, where the order in which the three numbers are multiplied does not affect the result, is called the associative property of multiplication.
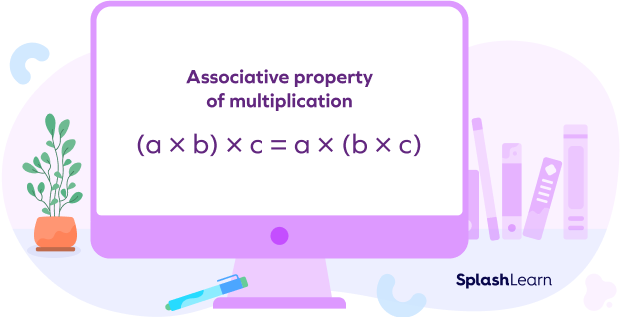
Since addition is the foundation of multiplication, the associative property is only followed by addition and multiplication. The law of associativity does not apply to the operations of subtraction and division.
Recommended Games
Solved Examples
Example 1: Solve the expression $6 \times 7 \times 8$ in two different ways.
Solution:
Grouping the first two terms in the expression,
$(6 \times 7) \times 8
$= (42) \times 8$
$= 336$
Grouping the second two terms in the expression,
$6 \times (7 \times 8)$
$= 6 \times (56)$
$= 336$
Example 2: Does the given equation show the associative property of multiplication?
$2 \times 3 \times 4 = 3 \times 2 \times 4$
Solution: For an equation to show the associative property of multiplication, a minimum of three numbers must be multiplied. The given equation is the multiplication of 3, 2, and 4. The order of the numbers reversed gives the same answer, that is, 24. Thus, it shows an associative property.
Example 3: Use associative property of multiplication to find a and b in the equation,
$(3 \times a) \times 9 = 3 \times (4 \times b)$
Solution: If the equation follows the associative property of multiplication, though grouped differently, the three terms on either side of the equation should be the same. 3 is present on either side. It follows that $a = 4$ and $9 = 6$.
Recommended Worksheets
Practice Problems
Associative Property of Multiplication - Definition With Examples
Which of the following does not show the associative property of multiplication?
The terms on the left are not the same as the ones on the right of the equation. The associative property is only valid for grouping the terms.
Given that $(10 \times m) \times 2 = 10 \times (5 \times 2)$, what is the value of m to make the equation true?
To make the equation true, it should follow the associative property of multiplication. The three terms on the left are 10, m, and 2, and on the right are 10, 5, and 2. Hence, clearly, $m = 5$.
If the following equation follows the associative property of multiplication, find p and q. $(8 \times p) \times 6 = 8 \times (11 \times q)
If the equation follows the associative property of multiplication, though grouped differently, the three terms on either side of the equation should be the same. 8 is present on either side. It naturally follows that $p = 11$ and $q = 6$, to ensure 8, 11, and 6 are present on either side as well.
Which of the following does not show the associative property?
The associative property applies to addition and multiplication but not to subtraction and division. The grouping of three or more terms multiplied or added does not affect the result.
Frequently Asked Questions
What is the benefit of using the associative property of multiplication?
The associative property of multiplication helps you multiply numbers faster. Instead of multiplying a list of numbers in the order in which they’re written, group them differently to multiply in an order convenient to you. In the expression $5 \times 5 \times (8)$, it would be wise to multiply 5 and 8 to get 40, then multiply 5 to get 200.
$(5 \times 5) \times (8)$
$= (5 \times 8) \times (5)$
$= 40 \times 5$
$= 200$
Apart from associative property, which other properties does multiplication follow?
The arithmetic operation of multiplication follows two other properties, which include commutative property and distributive property.
What is the difference between the commutative and associative property of multiplication?
The commutative property is concerned with the order of numbers, whereas the associative property is concerned with the grouping of numbers. In both cases, the result is not affected.
Commutative: $4 \times 5 = 5 \times 4$
Associative: $2 \times (4 \times 5) = 5 \times (4 \times 2)$
The commutative property can be applied to two terms. As more than two numbers are required for grouping, three or more terms must be present to apply the associative property.




































