Power of a Power Rule: Definition, Rules, Examples, Facts, FAQs

What Is the Power of a Power Rule?

The power of a power rule is an important exponent rule (law of exponent) used to simplify an expression of the form $(x^{m})^{n}$, where the base x is raised to a power m and the entire expression $x^{m}$ is raised to the power n again. 

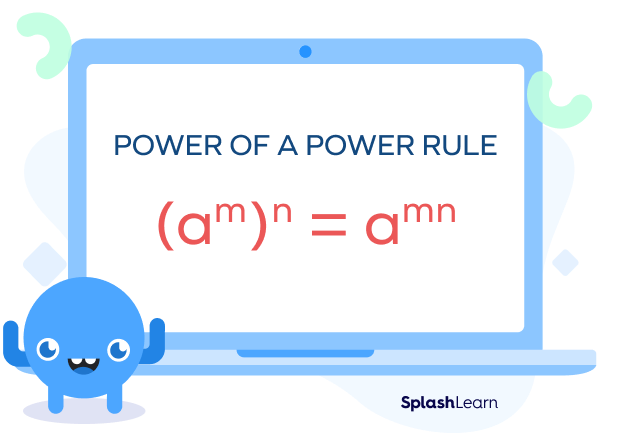
Power raised to a power rule is given by

$(x^{m})^{n} = x^{m \times n} = x^{mn}$ …where x is the base, m and n are exponents

This means that if a base is raised to power and on the whole term raised to another power, then the two powers are multiplied, keeping the same base.

• $x^{m}$ means that the base “x” is multiplied by itself  “m” times. 
• $(x^{m})^{n}$ means that the base $(x^{m})$ is multiplied by itself “n” times.
• $x^{mn}$ means the base “x” is multiplied by itself  “mn” times.

Power of a power rule examples:

• $(a^{2})^{3} = a^{2 × 3} = a^{6}$
• $(2^{4})^{2} = 2^{4 × 2} = 2^{8}$
• $\left[(p + q)^{5}\right]^{7} = (p + q)^{5 × 7} = (p + q)^{35}$
• $\left[(\;-\;1)^{4}\right]^{2} = (\;-\;1)^{4 × 2} =  (-1)^{8} = 1$

Power of a Power Rule with Negative Exponents

When we are dealing with the negative exponents, we have to also apply the multiplication rules used with respect to the negative sign. 

Note that $x^{-\;n} = \frac{1}{x^{n}}$.

• $(a^{-\;m})^{-\;n} = a^{-\;m ×\;-\; n} = a^{mn}$
• $(a^{-\;m})^{n} = a^{-\;m × n} = a^{-\;mn} = \frac{1}{a^{mn}}$
• $(a^{m})^{-\;n} = a^{m × \;-\;n} = a^{-\;mn}$
• $(x^{-\;m})^{-\;n} = x^{-\;m ×\;-\;n} = x^{mn}$

Examples: 

• $(2^{-\;2})^{-\;3} = (2)^{-\;2 × \;-\;3} = 2^{6} = 64$
• $(x^{-\;m})^{n} = x^{-\;m × n} = x^{-\;mn} = \frac{1}{x^{mn}}$
• $(2^{2})^{-\;3} = (2)^{2 × \;-\;3} = 2^{-\;6} = 164$

Fraction Power to Power Rule

Let’s understand the power to a power rule of exponents when the exponent is a fraction. 
In this case, the two denominators and numerators are multiplied.

The formula can be written as

• $(x^{\frac{m}{n}})^{\frac{p}{q}} = x^{\frac{pm}{qn}}$
• $(x^{m})^{\frac{p}{q}} = x^{\frac{pm}{q}}$
• $(x^{\frac{m}{n}})^{p} = x^{\frac{pm}{n}}$

Let us see some examples of rational power of a power rule to understand better:

• $(x^{\frac{1}{2}})^{4} = x^{\frac{1}{2} × 4} = x^{2}$
• $(2^{\frac{3}{2}})^{\frac{2}{3}} = 2^{\frac{3}{2} × \frac{2}{3}} = 2^{1} = 2$
• $(3^{-\;2})^{\frac{3}{2}} = 3^{-\;2 × \frac{3}{2}} = 3^{-\;3} = \frac{1}{3^{3}}$

Simplifying Expressions the Power of a Power Rule

Let’s simplify an expression using the power of a power rule.

Example: Find the value of $\left[(\;-\;3)^{2}\right]^{3}$.

To simplify the expression $(\;-\;3^{2})^{3}$, we have to apply the power to the power rule, because the base is raised to a power and the whole is raised to another power. So we have to multiply the powers 2 and 3 to simplify this expression.

$\left[(\;-\;3)^{2}\right]^{3}$

$= (\;-\;3)^{2 × 3}$

$=(\;-\;3)^{6}$

$= (\;-\;3) × (\;-\;3) × (\;-\;3) × (\;-\;3) × (\;-\;3) × (\;-\;3)$

$= 729$

Facts about Power of a Power Rule

Conclusion

In this article, we have learned the power of a power rule and its formula in detail. We have also understood the application of the power of a power rule in simplifying expressions with negative and rational exponents. Now let us solve and practice the rule with a few examples.

Solved Examples on Power of a Power Rule

1. Identify the value of $(5^{2})^{2}$.

Solution:

Given expression: $(5^{2})^{2}$

The power of a power formula is, $(a^{m})^{n} = a^{mn}$

Let us multiply both the powers,

$(5^{2})^{2} = 5^{2 × 2} = 5^{4}$

Now solve the expression

$5^{4} = 5 × 5 × 5 × 5$

$5^{4} = 625$

Thus the value of the expression  $(5^{2})^{2}$ is 625.

2. Find the value of $\left[(\;-\;3)^{-\;3}\right]{-\;2}$.

Solution:

Given expression: $\left[(\;-\;3)^{-\;3}\right]^{-\;2}$

By using the power of a power formula, $(a^{-\;m})^{-\;n} = a^{-\;m × \;-\;n} = a^{mn}$

Let’s solve the expression.

$\left[(\;-\;3)^{-\;3}\right]^{-\;2}$

$= (\;-\;3)^{-\;3 × \;-\;2}$

$= (\;-\;3)^{6}$

$= 729$

Thus the value of the expression $\left[(\;-\;3)^{-\;3}\right]^{-\;2}$ is 729.

3. Find: $\left[(125)^{6}\right]^{\frac{1}{2}}$.

Solution:

Formula: $(x^{m})^{\frac{p}{q}} = x^{\frac{pm}{q}}$

$\left[(125)^{6}\right]^{\frac{1}{2}}$

$= (125)^{6 × \frac{1}{2}}$

$= (125)^{3}$

$= 5$

Practice Problems on the Power of a Power Rule

Frequently Asked Questions on the Power of a Power Rule