Properties of Equality – Definition, Examples, Applications, FAQs

What Are the Properties of Equality?

Properties of equality are fundamental rules that apply to equations and express the idea that both sides of an equation are equal. If an arithmetic operation has been used on one side of the equation, then the same should be used on the other side. Properties of equality are all about balance. 

The properties of equality for real numbers consist of several fundamental rules.  The properties of equality of real numbers: 

(i) Addition Property of Equality (ii) Subtraction Property of Equality (iii) Multiplication Property of Equality  (iv) Division Property of Equality (v) Reflexive Property  (vi) Symmetric Property (vii) Transitive Property (viii) Substitution Property  (ix) Square Root Property

While some textbooks may only include the first 8 properties of equality, others cover all the properties. These rules govern how equations can be manipulated and help ensure that both sides of an equation remain equal throughout various operations and calculations.

These properties allow us to manipulate equations, perform operations, and solve for unknown variables in a consistent and reliable manner. Understanding and applying these properties is essential in algebra and other branches of mathematics.

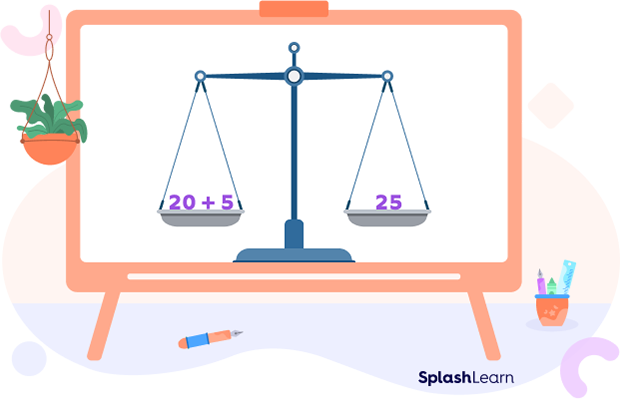
Properties of Equality Definition

The properties of equality are distinctive characteristics that have no impact on the truth of an equation. They maintain the equality of two or more values during various operations. These properties can create equivalent arithmetic or algebraic equations and facilitate solving equations based on equality properties.

List of Different Properties of Equality

Let’s discuss each property of equality one by one.

Addition Property of Equality

When you add the same value to both sides of an equation, the equation remains true. This concept is known as the addition property of equality. 

Mathematically, for real numbers a, b and c, we have

If $a = b$, then $a + c = b + c$.

Subtraction Property of Equality

When you subtract a real number from both sides of an equation, the equation remains true. This principle is known as the subtraction property of equality. 

To understand this mathematically, let’s assume that p, q and r are real numbers.

If $p = q$, then $p \;-\; r = q \;-\; r$

Multiplication Property of Equality

When you multiply both sides of an equation by the same real number, the equation remains balanced. This is known as the multiplication property of equality.

Let’s understand this mathematically. Consider any three real numbers, a, b and c.

If $a = b$, then $a \times c =  b \times c$

Division Property of Equality

 If you divide both sides of an equation by the same non-zero real number, the equation remains equal. This is known as the division property of equality.

Let’s assume three real numbers, x, y and z.

If $x = y$ and $z \neq 0$, then according to the division property of equality,

$\frac{x}{z} = \frac{y}{z}$

Reflexive Property of Equality

The reflexive property of equality states that each real number is always equal to itself.

For any real number x, x = x.

Symmetric Property of Equality

By symmetric property of equality, the order of equality does not matter.

For all real numbers x and y, if x = y , then y = x.

Transitive Property of Equality

By the transitive property of equality, two numbers that are equal to the same number are also equal.

If p, q and r are real numbers such that p = q and q = r, then p = r.

Substitution Property of Equality

According to the substitution property of equality, if we have two real numbers equal to each other, then we can substitute the value of any of them in any algebraic equation. 

For all real numbers x and y, if x = y, then we can substitute y for x in any expression.

Square Root Property of Equality

This property of equality states that if two real numbers are equal, their square roots will also be equal. 

To better understand, let’s see it mathematically

Let x and y be two real numbers

If x = y, then $\sqrt{x} = \sqrt{y}$

Table for Properties of Equality

Now we have understood each property of equality in detail. Let us create a simplified table for the properties of equality equations to help us have a quick revision of all of them. Let a, b and c be real numbers.

Property of Equality	Mathematically Meaning
Addition property of equality	If a = b, then $a + c = b + c$.
Subtraction property of equality	If a = b, then $a \;-\; c = b \;-\; c$.
Multiplication property of equality	If a = b, then $a \times c = b \times c$.
Division property of equality	If a = b and $c \neq 0$, then $\frac{a}{c} = \frac{b}{c}$
Reflexive property of equality	a = a
Substitution property of equality	If a = b, then b can substitute a in any algebraic expression.
Transitive property of equality	If a = b and b = c, then a = c.
Symmetric property of equality	If a = b, then b = a.
Square root property of equality	If a = b, then $\sqrt{a} = \sqrt{b}$.

Applications of Properties of Equality in Math

• Solving equations using properties of equality: The properties of equality are essential in solving equations. By applying these properties, we can manipulate the equations to isolate the unknown variable and find its value.
• Simplifying Expressions: When simplifying algebraic expressions, the properties of equality help in combining like terms and reducing complex expressions into simpler forms.
• Proving Theorems: In mathematical proofs, the properties of equality are often used to demonstrate the equality of different expressions or values, providing a logical basis for mathematical statements.
• Real-life uses: Properties of equality are frequently applied in everyday situations without us realizing it. Imagine you have a recipe that serves 4 people, and you want to adjust it to serve 8 people for a party. You can use the multiplication property of equality to do this. By multiplying the ingredients in the recipe by 2 (since 8 is twice the number of people as 4), you can easily double the quantity of each ingredient and make enough food to serve 8 people.

Now, let’s understand their application using some properties of equality examples. 

Example 1: Find the value of x in the following expression: 

(i) 3 + x = 15 

(ii) 4x = 48 

(iii) x – 5 = 72

Solution:

(i) 3 + x = 15

Subtract 3 from both sides of the equation.

$\Rightarrow 3 + x \;-\; 3 = 15 \;-\; 3$ Subtraction property of equality

$\Rightarrow x = 15 \;-\; 3$

$\Rightarrow x = 12$

(ii) 4x = 48 

Dividing both sides of the equation by 4.

$\Rightarrow \frac{4x}{4} = \frac{48}{4}$ Division property of equality

$\Rightarrow x = \frac{48}{4}$

$\Rightarrow x = 12$

(iii) x – 5 = 72

Add 5 on both sides of the equation.

$\Rightarrow x – 5 + 5 = 72 + 5$ Addition property of equality 

$\Rightarrow x = 72 + 5$

$\Rightarrow x = 77$

Facts about Properties of Equality

Conclusion

In this article, we learned about the properties of equality, fundamental principles that govern the manipulation of equations and real numbers. Understanding these properties is crucial for algebraic manipulations and solving equations. Now, let’s solve a few examples and practice MCQs to reinforce our understanding and boost our confidence in working with equations and inequalities. Happy problem-solving!

Solved Examples on Properties of Equality

Example 1: Find the value of the expression x³ + x² + x – 4 if x = 2.

Solution:

By the Substitution property of equality, if a = b, then b can substitute a in any algebraic expression.

Given expression: x³ + x² + x – 4

Substitute the value of x = 2 in the expression.

2³ + 2² + 2 – 4

= 8 + 4 + 2 – 4

= 12 + 2 – 4

= 14 – 4

= 10

Example 2: Solve the following.

(i) 3x – 6 = 30 

(ii) $\frac{5}{2}x + 8 = 58$

(iii) 13x + 5 = 96

Solution:

(i) 3x – 6 = 30

Using the Addition property of equality, we add 6 on both sides of the equation.

$\Rightarrow 3x \;-\; 6 + 6 = 30 + 6$

$\Rightarrow 3x = 36$

Now using the Division property of equality, we divide both sides of the equation by 3.

$\Rightarrow \frac{3x}{3} = \frac{36}{3}$

$\Rightarrow x = 12$

(ii) $\frac{5}{2}x$ + 8 = 58

Using the Subtraction property of equality, we subtract 8 from both sides of the equation.

$\frac{5}{2}x$ + 8 – 8 = 58 – 8

$\frac{5}{2}x$ = 50 ————– (1)

Use the Multiplication property of equality, we multiply equation (1) by $\frac{2}{5}$. 

$\Rightarrow \frac{5}{2}x \times \frac{2}{5} = 50 \times \frac{2}{5}$

$\Rightarrow x = 10 \times 2$

$\Rightarrow x = 20$

(iii) 13x + 5 = 96

Using the Subtraction property of equality, we subtract 5 from both sides of the equation 

$\Rightarrow 13x + 5 \;-\; 5 = 96 \;-\; 5$

$\Rightarrow 13x = 91$

Using the Division property of equality, we divide both sides by 13.

$\Rightarrow \frac{13x}{13} = \frac{91}{13}$

$\Rightarrow x = 7$

Example 3: Julie bought some oranges from the market. Three-fourth of the total oranges were rotten. If the number of rotten oranges is 36. What was the total number of oranges Julie bought?

Solution:

Let the total number of oranges be n. 

$\frac{3}{4}$ of the total number = 36

$\Rightarrow \frac{3}{4} \times n = 36$

$\Rightarrow \frac{3n}{4} = 36$

Now, using the Multiplication property of equality, we multiply both sides by 43.

$\Rightarrow \frac{3n}{4} \times \frac{4}{3} = 36 \times \frac{4}{3}$

$\Rightarrow n = 12 \times 4$

$\Rightarrow n = 48$ 

Therefore, the total number of oranges that Julie bought was 48 oranges.

Practice Problems on Properties of Equality

Frequently Asked Questions about Properties of Equality